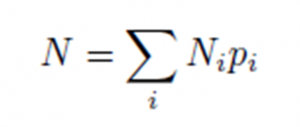Astrobites RSS

galaxy disruption

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Empirical Determination of Dark Matter Velocities using Metal-Poor Stars
Authors: Jonah Herzog-Arbeitman, Mariangela Lisanti, Piero Madau, Lina Necib
First Author’s Institution: Princeton University
Status: Submitted to ApJL

Our galaxy is embedded in a cloud of dark matter, thought to consist of tiny particles traveling along orbits through the halo. These dark matter particles permeate all regions of the galaxy, extending far beyond the edge of the bright central spiral, but also orbiting through our solar system, and even passing right through the Earth. This is why scientists build giant detectors, hoping to trap some of these dark matter particles as they pass by. So far, these experiments have not detected dark matter, but that lack of detection is actually quite interesting. Finding out what dark matter is not, and thereby narrowing down the possibilities, is an important step towards revealing the true nature of these mysterious particles.

In order to really understand what it means when a detecter does not see dark matter, it is important to have a clear prediction for how much dark matter should be detected. For example, if we expect very few dark matter particles to pass through the Earth in a given amount of time, then maybe the lack of detections over a few years doesn’t actually mean those particles don’t exist. One essential piece of information in this prediction is the velocity of dark matter particles as they orbit past our solar system.

So, how can we determine the speed of these particles that we haven’t even directly detected? Well, let’s look back at where these particles actually come from. Dark matter halos grow over time by consuming other dark matter halos. This process is called hierarchical structure formation. The Milky Way is continuously pulling in smaller galaxies and then tearing them apart, thoroughly mixing their stars and dark matter particles into the Milky Way halo (Figure 1).

This understanding of the origin of these particles reveals an important piece of information: when dark matter particles join the Milky Way, they are often accompanied by stars. This is great news, because stars, unlike dark matter particles, are not invisible, and we can directly measure their velocities. If we can confirm that dark matter particles tend to move at similar velocities to their stellar companions, then this problem of determining the local dark matter velocity is much simpler! Finding out if this is in fact the case is exactly the goal of today’s paper.

The tricky thing here is that the Milky Way is continuously forming new stars, so the authors need to find a way to distinguish between the stars formed within the Milky Way and stars that formed in smaller galaxies and were then consumed by the Milky Way along with the corresponding dark matter. This turns out to be fairly straightforward: stars that form in smaller galaxies tend to have a different chemical composition than stars that are currently being formed in the Milky Way. This is because Milky Way stars are forming from materials that have been enriched with heavier elements by generations of star formation, while the stars in smaller galaxies are not. The stars we are interested in are therefore what astronomers call “metal-poor.” The prediction is therefore that metal-poor stars and dark matter particles should have similar velocities.

Figure 2. A simulated Milky Way-like galaxy, from the ERIS simulation used in this paper. [Simone Callegari]

The authors use simulations of Milky Way-like galaxies (Figure 2) to compare the velocities of dark matter and stars, and find that this prediction holds up! Figure 3 shows the distributions of velocities for dark matter and different stellar populations. The black histogram is dark matter, the cyan histogram is all stars, and the orange histogram is only metal-poor stars. The black and orange histograms line up pretty well, meaning the velocity of metal-poor stars does tend to match that of the dark matter. This means that by observing the velocities of these stars near the Sun, we can improve our understanding of the dark matter velocity. This will improve our interpretation of the results of dark matter experiments. In particular, based on preliminary calculations, the authors show that the velocity is lower than previously thought. They suggest that this may weaken the significance of non-detections at smaller dark matter particle masses.

Figure 3. Velocity histograms of different components of the Milky Way, as seen in the ERIS simulation. The black histogram shows the velocity distribution of dark matter. The cyan histogram illustrates the velocity of all stars, and has a much larger central peak than the dark matter distribution. The orange histogram, however, which includes only metal-poor stars, is very similar to the dark matter velocity distribution. [Herzog-Arbeitman et al. 2018]

This is a really exciting result. Previous estimates of the dark matter velocity all came from simulations and theoretical predictions, so this new method, which uses observations of our actual galaxy, rather than a simplified model, should really improve the accuracy of these calculations. Furthermore, current experiments like Gaia are greatly improving our understanding of the local stellar velocity distribution, which will continue to increase the power of this method to determine the local dark matter velocity.

About the author, Nora Shipp:

I am a 2nd year grad student at the University of Chicago. I work on combining simulations and observations to learn about the Milky Way and dark matter.

dwarf galaxy

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Hunting Faint Dwarf Galaxies in the Field Using Integrated Light Surveys
Authors: S. Danieli, P. van Dokkum, C. Conroy
First Author’s Institution: Yale University
Status: Submitted to ApJ

One marvelous fact about our universe is that at the largest scales, it is fractal. Unlike true fractals, which exhibit exact self-similarity, the universe is only statistically self-similar. If you looked at the most massive objects in the universe, a record held by the gargantuan, invisible dark matter blobs holding clusters of galaxies together, which clock in with masses upwards of 1015 times the mass of the Sun, you’d find that they’re rife with smaller blobs, or “halos” of dark matter. Many of these smaller dark matter halos are inhabited by a galaxy, including giant bright elliptical galaxies, the smaller and fainter spiral galaxies, and hordes of yet smaller, fainter galaxies. Peering closer at, say, one of the dark matter halos of a Milky Way-like spiral galaxy, which clocks in at about 1012 times the mass of the Sun, you’d find that it in turn is surrounded by a similar but down-sized army of even smaller dark matter halos, which may contain even fainter “dwarf” galaxies. And the halos of each of these dwarf galaxies in turn can host their own army of even tinier dark matter halos. If you just looked at the dark matter of a galaxy cluster, a single spiral galaxy, or a dwarf galaxy, it would be hard to tell which was which — they would roughly look like scaled up (or down) versions of each other.

How far down does this fractal structure go? We can search part of the way down by searching for the smallest, faintest galaxies that live within them — which is an incredibly difficult task. To go further down to the smallest dark matter halos, which may be completely dark, and thus unobservable by usual means (i.e. by light), we’ll have to turn to more exotic methods. The faintest dwarf galaxies we’ve found thus far have been discovered by hunting for clusters, or “overdensities,” of stars. This technique can only uncover dwarfs in which we can observe individual stars, which we can distinguish only out to a pitiful distance — just to up to about 5 Mpc away, which is a little beyond the edge of the Local Group of neighborly galaxies.

What about the faint dwarfs that live even further away, far enough that they appear as fuzzy patches of light, and not as collections of stars? The authors of today’s paper discuss our prospects for finding these “integrated light” images of dwarfs (see Fig. 1) as far away as 10 Mpc. To do this, they set out to ask a simple question: how many galaxies could they find, given a telescope with a particular resolution and sensitivity?

Figure 1. Simulated observations of a faint dwarf galaxy at different distances from the Milky Way. If the dwarf is just outside the Milky Way, at about 500 kpc, we can see the individual stars within the galaxy. However, if such a galaxy is farther away, it becomes increasingly difficult to resolve individual stars. At 4 Mpc, it only appears as a fuzzy blob of light, and we can no longer see the individual stars in the galaxy. Detecting such faraway, faint dwarfs requires new search methods. [Danieli et al. 2017]

To carry out this calculation, the authors assume — for lack of data — that the faint galaxies as far as 10 Mpc look like the ones we’ve seen that are close by. Based on these nearby galaxies, they estimate how large and faint the distant dwarfs they’re searching for would be, then determine whether or not we could see them. They also estimate the mass in stars each of these faraway dwarfs have — a key quantity that allows them to guess the mass of the dark matter halos the dwarfs inhabit. It’s as yet unclear how much dark matter a galaxy with a given mass in stars has — a mapping we call the stellar mass-halo mass (SMHM) relation — so the authors adopt two different ones. With the dark matter mass of the dwarf galaxies, it’s simple enough to determine the number of such dwarfs that should exist out to 10 Mpc from simulations of the Milky Way and its surroundings.

Figure 2. The number of dwarfs between 3–10 Mpc that we could see with a telescope of a given resolution and sensitivity. The angular resolution is shown on the horizontal axis, and the sensitivity, here quantified as the surface brightness μ, is shown on the vertical axis. The colors and black contour lines denote the number of dwarfs you can see per square degree in the sky (an area equivalent to five times the Moon’s). The two panels show results from two different SMHM relations (see above paragraph for details). Brighter dwarf galaxies — those with smaller μ and thus at the bottom of the plots — can be seen no matter the resolution of your telescope. Fainter dwarfs — those with larger μ and higher up on the plot — are found in greater abundance, and we need good spatial resolution (fewer arcsecs) to detect them all. [Danieli et al. 2017]

The authors find (see Fig. 2) that, as you might expect, brighter dwarfs can be discovered no matter how good the resolution of your telescope is. However, when attempting to discover fainter dwarfs, the resolution really begins to matter. If, say, you had a telescope with a resolution of 9 arcsec versus one that was twice as good, you could detect up to six times as many of the faintest dwarfs. They also find that the SMHM they assume can affect the number of the faintest dwarfs they expect to find by as much as a factor of five.

The authors calculate that using this “integrated light” method to hunt for faint dwarfs using the Dragonfly Telescope Array, a telescope that was designed for the task, we could find a similar number of galaxies — if not more — as with surveys that rely on the traditional method of finding clusters of individually resolved stars. This is an exciting result. The few smallest, faintest galaxies we’ve found so far currently puzzle astronomers: how many of them are there? Why are they so faint? How did their stars form? We could begin to unravel these mysteries once we find more of these tiny galaxies.

About the author, Stacy Kim:

I am a fourth-year graduate student in The Ohio State University’s Department of Astronomy. On a day-to-day basis, you can typically find me attempting to smash clusters of galaxies together inside big supercomputers with Dr. Annika Peter to see if cluster mergers are good testbeds for dark matter collisionality. As an undergraduate at Caltech, I spent a few years chasing photons where planets are thought to form (or, as they say, performing Monte Carlo radiative transfer calculations of protoplanetary disks) with Dr. Neal Turner of the Jet Propulsion Laboratory. When I’m not sitting in front of a computer trying to translate cosmic thoughts into pithy lines of code, you can find me in the kitchen or on the walls of a climbing gym.

Icy exoplanet

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Towards a Galactic Distribution of Planets. I: Methodology & Planet Sensitivities of the 2015 High-Cadence Spitzer Microlens Sample
Authors: Wei Zhu, A. Udalski, S. Calchi Novati et al.
First Author’s Institution: Ohio State University
Status: Published in AJ

I don’t know if you’ve heard, but astronomers have found quite a few exoplanets in the last couple of decades. However, most of these are clustered in our tiny corner of the galaxy. For the 2,043 planets with stellar distance listed on exoplanets.org today (yes, I know this article will be out of date in a week…) the average distance from to the host star from Earth is 624 pc. The center of the galaxy, meanwhile, is ~8,000 pc away. That’s further than even the furthest known exoplanet, OGLE-05-390L b, which is 6,500 pc from us.

And we’d really like to have a better understanding of the exoplanets in the galactic bulge, because their presence — or lack thereof — helps us to understand planet formation. Planet formation is believed to be affected by several external factors such as the host star’s metallicity, the stellar mass, the stellar multiplicity, and the stellar environment. That final category is what we’re going to consider today: does the presence of a large number of nearby stars interrupt the formation of planets? The galactic bulge, as the part of the galaxy with the highest number density of stars, is an ideal place to test this — if only we could detect enough planets out there…

OGLE telescope at the Las Campanas Observatory in Chile. [Krzysztof Ulaczyk]

Any readers particularly clued-up on exoplanet surveys might have recognised the phrase ‘OGLE’ in the name of planet ‘OGLE-05-390L b’. OGLE is the Optical Gravitational Lensing Experiment, a microlensing project run by Warsaw University. Although the mission was initially designed for dark-matter surveys, it has also made several serendipitous exoplanet discoveries. This astrobite describes microlensing for exoplanet detection in more detail, but for today all we really need to know is that sometimes nearby stars and distant stars happen to be really well aligned on the sky for a short time. In these cases, the nearby star’s gravity bends the light from the faraway star, causing it to be brighter for a short time; this is the process we call microlensing. If the nearby star also has a planet, which is also well aligned with the distant star, then the gravitational influence of the planet plus star system causes the brightness of the microlensing event to vary in a particular way. The planet/star mass ratio can be inferred from the precise shape of that brightness plot. This is, of course, the same physics that produces the stunning, strong-lensing Einstein Rings — but with a slightly weaker requirement for close alignment.

Unlike the radial-velocity, transit or direct-imaging methods for exoplanet hunting, the microlensing technique is able to detect exoplanets at huge distances. Meanwhile, a field with physically more stars is a great place for microlensing experiments, since a large number of stars need to be monitored for a long time so as to catch some of these chance alignments of foreground and background stars. As such, OGLE has been staring at the center of the galaxy for over a decade.

More recently, the microlensing community has become particularly interested in microlensing detections that have been measured by multiple different telescopes simultaneously. In a typical microlensing event, the mass and the distance of the foreground lensing star are degenerate. However, this degeneracy can be broken by comparing several simultaneous observations of the microlensing event with physically separated telescopes. The wider the separation between the different telescopes measuring the microlensing detection, the better — so why not use a telescope in space? The Spitzer telescope is almost 200,000,000 km away, giving an impressive distance baseline for this kind of work.

Figure 1: Sensitivities to planets for a subset of the survey. Red, green, blue, purple and black curves show the depth to which 15, 30, 45, 60 and 75% of planets could be detected; q and s represent the mass ratio and projected separation of the planets. The bottom left corner lists the OGLE catalog number (bold) and the impact parameter, which represents the closest on-sky separation of the two stars during the entire event. [Adapted from Zhu et al. 2017]

Today’s authors carry out a pilot study laying out the methodology for a microlensing survey exploring how the galactic bulge affects planet formation. After data validation, removal of instrumental systematics, and a check that the distance to each star is well defined, the authors use a sample of 41 microlensing events — all of which have been observed by OGLE, Spitzer and a third telescope, KMTNet. Each of these microlensing events consists of a distant and a nearby star — and in this case no planets are detected around the nearby stars.

The authors model each of the lensing events with a variety of planets orbiting the nearby star, so as to determine how sensitive the survey is to exoplanets as a function of mass and separation. Some of these sensitivity curves are shown in Figure 2 above. The authors then carry out a statistical analysis; for this they use a simple parametrised model of the galaxy as a bulge and a disk, a couple of different assumptions about the stellar mass function, the footprint of the survey on the sky, some beastly Bayes calculations and information about their survey’s sensitivity to planets.

On the assumption that planetary frequency is the same in the galactic bulge, the authors find that roughly a third of all planetary detections in a survey like this one should come from bulge events. Since they have no planet detections in this sample, they aren’t yet able to calculate what fraction of detections actually come from the galactic bulge — this is left as future work. If the number turns out to be significantly different from the value of one third calculated here, it will reveal crucial information about planet formation in crowded regions of the galaxy. This work is currently ongoing. And, if they get lucky, maybe OGLE-05-390L b’s record as most-distant-planet will soon be broken!

About the author, Elisabeth Matthews:

I’m a third year PhD student at the University of Exeter, in the south of England, where I’m aiming to detect and characterise extrasolar planets and debris disks via direct imaging. So far this has meant lots of detecting background stars that happen to be well aligned with bright, nearby stars and no detecting of actual planets — but hopefully my luck will change soon!

Proxima Centauri

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: ALMA Discovery of Dust Belts Around Proxima Centauri
Authors: Guillem Anglada, Pedro J. Amado, Jose L. Ortiz, and collaborators
First Author’s Institution: Institute of Astrophysics of Andalusia, Spain
Status: Published in ApJL

Hiding behind the gleaming light of a pale red dot lies one of the world’s favorite exoplanets, Proxima b, whose orbital motion imprints a barely detectable wobble in its host star, Proxima Centauri. Following the seminal discovery of a planet in its habitable zone, this undistinguished low-mass red dwarf star became a target for tireless examination, despite the challenges involved in observing it. In today’s paper, we will see that one of these searches, however, seems to have paid off … in dust?

Figure 1. Observations of Proxima Centauri with the ALMA observatory suggest that it may not only host a rocky planet but also at least one dust ring around it. [Anglada et al. 2017]

The One Ring

Okay, I will be honest and admit that, indeed, few people in the world would be enthusiastic about dust — in space, of all things! But, let me tell you, this is exciting dust. Guillem Anglada and his collaborators (including his spacefaring self from a parallel universe, Guillem Anglada-Escudé, who is the first author of Proxima b’s discovery paper) used the Atacama Large Millimeter Array (ALMA) to observe a dust ring around Proxima Centauri. Such an object is a signpost of terrestrial planet formation and could clue us in on what its planetary system looks like and how it came to be.

You may recall that the lore of Middle Earth tells the story of The One Ring to Rule Them All™, whose (un)fortunate wearer is granted the power to become invisible. In a funny exchange of roles, the dust ring around Proxima Centauri may actually help us uncover more information about the well-hidden planet Proxima b, such as its orbital inclination and mass.

But leaving western epic novels aside, let’s talk about the instrument. The ALMA observatory has two types of antennas: the smaller 7-meter diameter detectors, and the bigger 12-meter ones. The data that comes from these antennas differ in that the smaller ones produce images with fewer details but with a wider field of view; the bigger antennas produce more detailed images but see a limited field of view.

Following analysis of the data depicted in Figure 2 below, the authors found that Proxima Centauri appears to emit more infrared light than it should, which they conclude must be produced by a “belt,” or ring, of cold (50 K) dust. The dust belt has a radius four times the distance of the Earth from the Sun (i.e. 4 AU). In reality, this dust ring seems to be an analog to the Solar System’s Kuiper Belt. They estimate that the mass contained in this belt is 1% that of Earth’s, which is similar to the mass of the Kuiper Belt around the Sun.

Figure 2. Image of Proxima Centauri (represented by the + mark) using ALMA’s 12-meter array. Although not clearly separated from the central source, the observed infrared excess strongly suggests the presence of a dust belt around the star with a radius smaller than 4 AU. Its shape also hints at the presence of a warmer belt closer to the central star, but this hypothesis needs confirmation. The identity of the detached turquoise blob to the left of the star is unknown: it could be either a real object or plain noise fluctuation (see main text). [Anglada et al. 2017]

Rings for Days

In addition to the “One Ring” described above, the elongated shape of the source in Figure 2 suggests the presence of a warmer (T ~ 90 K) dust belt with size 0.8 AU, but the authors don’t seem to be completely sure about its nature yet.

Now, if you’re asking yourself what that blob of emission to the lower left of Proxima Cen in Figure 2 could possibly be, the short answer is: we don’t know yet. The long answer is that it could either be a real source or it could just be random noise fluctuation. If it is a real source then the authors suggest many possible explanations, such as a background galaxy or a collision between large bodies. But the most intriguing explanation for this potential source is that we could be looking at the rings of a Saturn-like planet orbiting Proxima Cen. More observations will be needed to confirm this exciting possibility, though.

But wait, there’s more! Figure 3 below depicts the image as observed by the compact array configuration of ALMA, using the 7-meter antennas: what seems to be a second dust belt is seen as a series of green smudges at a distance of approximately 30 AU, represented by the white ellipse. Although the detection is very marginal, the authors propose that this could be an outer dust ring with 1/10,000 the mass of Earth.

Figure 3. Image of Proxima Centauri using ALMA’s compact array configuration (7-meter antennas). The dotted white ellipse marks the position of a dust belt of radius 30 AU. The green regions are the positions where the signal produced by the dust belt is stronger.

I know, I know, that was a lot to take in! Proxima Centauri suddenly seems a lot busier than we previously thought, likely sporting a rocky planet in its habitability zone and a Kuiper Belt-analog. The other possibilities, such as the Saturn-like ringed planet and the other dust belts are still a bit speculative, so further observations of the system are a no-brainer at this point.

About the author, Leonardo dos Santos:

Leo is an exoplanet scientist and Ph.D. candidate at the Geneva Observatory. His current research involves characterization of exoplanets, physical and chemical properties of stars similar to the Sun and developing astronomical software. Not to be confused with the constellation.

turbulent disk

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: The Effects of Protostellar Disk Turbulence on CO Emission Lines: A Comparison Study of Disks With Constant CO Abundance vs. Chemically Evolving Disks
Authors: Mo (Emma) Yu, Neal Evans, Sarah Dodson-Robinson, Karen Willacy, Neal Turner
First Author’s Institution: University of Texas at Austin
Status: Accepted to ApJ

We know it happens. We see that protoplanetary disks — the birthplace of planets — spill the gaseous material at their inner edge onto the young stars around which they orbit. This process of accretion persists throughout the disk’s lifetime and within 1 to 5 million years (or 10 Myr in rare cases), a disk will feed all of its gas to its star and completely fade away — leaving behind only the planets that formed along the way and any leftover rocky material that remains.

The rate at which accretion occurs — as well as why accretion occurs — are both driving forces behind determining what types of planets can form quickly enough in a protoplanetary disk’s relatively short lifetime. While we can measure accretion rates onto stars directly, we still do not know why disks accrete! This is one of the most important unsolved problems in planet formation. Until we can solve it, our models for planet formation are incomplete.

There are two leading potential explanations for why accretion occurs: (1) turbulence, and (2) magnetic winds. In the last two years, several attempts have been made to measure turbulence in a nearby disk for the first time using CO (carbon monoxide) spectral lines. These measurements showed that the turbulence in that system is not strong enough to be responsible for accretion, winning favor for the other idea of magnetic winds.

However, today’s paper led by Emma Yu argues that those measurements were not interpreted properly since they did not take into account the relatively rapid rate at which CO depletes over time (see Figure 1). This paper asks: What can we really learn about turbulence from CO spectral lines if we include CO depletion in our model?

CO depletion

Figure 1: Density of CO and H2 at a range of distances from the star over time (taken from chemical models). Previous measurements of turbulence assumed the ratio of CO-to-H2 to be constant over time, but this is not true beyond 15 AU after 1 Myr. [Adapted from Yu et al. 2017]

Background: Why Do Protoplanetary Disks Accrete onto Their Stars?

It is understood that disks must accrete by transporting angular momentum outwards. Naively, we might expect this to happen through “viscous shear flow.” In this process, the gaseous disk struggles to behave like a fluid because it rotates at a slower rate further away from the star (due to Kepler’s 3rd law). As a result, material in the outer disk will try to speed up to catch up to the inner disk — thereby increasing its angular momentum. Meanwhile to compensate for that increase (and conserve angular momentum), the material in the inner disk will slow down. Since this gas is rotating too slowly to obey Kepler’s 3rd law, it will spiral inwards and eventually accrete.

There is just one problem: disks are too sparse to interact viscously like a fluid! For shear flow to occur, there must be some source of turbulence to make the disk viscous. It is widely accepted that magnetic fields can create turbulence by triggering an instability, but it is not clear if this instability actually occurs in the entire disk. If it does not occur in most of the disk, turbulence most likely would not be responsible for making the disk accrete.

Instead, magnetic winds — which transport angular momentum outward in a completely different way by flinging material out of the disk on magnetic field lines pointed away from the star while the disk rotates (see also here and here for more) — would be the favored reason for the disk accreting.

Introduction to Spectral Lines with Turbulence

We would know why protoplanetary disks accrete if we could just measure their levels of turbulence, but we did not have the telescope power to do this until the ALMA telescope array was turned on several years ago. In this astrobite, Tim discussed a new method for measuring turbulence with ALMA by looking at the shape of a specific CO spectral line.

Spectral lines are one of the most important tools in astronomy. They are used for everything from inferring compositions of atmospheres to measuring distances to distant galaxies, among many other things. Molecules (and atoms) emit spectral lines when electrons that were excited to a high energy state transition back to a lower energy state. While these lines are emitted at the precise wavelength corresponding to a transition, they never appear perfectly thin. They always exhibit some level of “broadening” due to the gas molecules moving towards us or away from us as they emit.

The velocities of individual molecules are mostly random. Thermal effects create most of the random motion for gas molecules. Besides that, turbulence also creates a little bit of random motion, causing spectral lines to broaden more than normal. As a result, we can use the broadened shapes of spectral lines (that are very well-resolved) to measure turbulence!

Spectral Lines with CO Depletion

The paper covered in Tim’s astrobite found that turbulence can be probed by measuring the CO spectral line’s peak-to-trough ratio (see Figure 2). Specifically, disks that are less turbulent have higher peak-to-trough ratios. However, this analysis assumed that the amount of CO in the disk relative to hydrogen is fixed over time, whereas chemical models predict it should actually drop significantly in older disks (see Figure 1).

CO spectral line profiles

Figure 2: CO spectral line profiles with different levels of turbulence, with lower levels producing a higher peak-to-trough ratio. (Note: The double-peak structure arises from part of the disk rotating towards us and part of the disk rotating away from us.) [Adapted from Yu et al. 2017]

Coincidentally, Emma Yu et al. find that the depletion of CO also creates spectral lines with higher peak-to-trough ratios (see Figure 3). As a result, the authors of the other study may have thought that they detected low levels of turbulence by measuring a high peak-to-trough ratio when they may really only be seeing evidence that CO has been depleted!

two disk stages

Figure 3: CO spectral line profiles at different stages in the disk lifetime. Left: Includes CO depletion. Right: Assumes constant CO-to-H2 ratio. With a constant CO ratio, the peak-to-trough ratio does not change after 1 Myr. With CO depletion, the peak-to-trough ratio increases over time just like with decreasing levels of turbulence. [Adapted from Yu et al. 2017]

Figures 2 and 3 (and also Figure 7 of the paper) show how easy it is to confuse a disk with no turbulence at all with a disk that has a moderate level of turbulence. If that extra turbulence is present, it might be strong enough to explain the observed levels of accretion in the previous study, giving favor to the long-assumed idea that turbulence drives accretion — not magnetic winds. However, the paper shows that our current measurements may not be able to distinguish these levels of turbulence, leaving the question of why disks accrete still unsolved.

Future Work

All hope is not lost! The authors point out that a different isotopologue of carbon monoxide (C18O) may be more useful for measuring turbulence. However, its spectral lines are weaker and thus, more difficult to resolve. They also point out that CO would be more helpful if we could also measure the precise level of CO depletion in a disk (rather than infer it from chemical models).

This is an exciting time for studying protoplanetary disks because we are finally beginning to scratch the surface of measuring turbulence. Getting proper measurements though, will require more digging.

About the author, Michael Hammer:

I am a 3rd-year graduate student at the University of Arizona, where I am working with Kaitlin Kratter on simulating planets, vortices, and other phenomena in protoplanetary disks. I am from Queens, NYC; but I’m not Spider-Man…

Aurorae on Jupiter

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: The Detectability of Radio Auroral Emission from Proxima b
Authors: Blakesley Burkhart & Abraham Loeb
First Author’s Institution: Harvard-Smithsonian Center for Astrophysics
Status: Published in ApJL, open access

Dazzling auroral displays are not uncommon in our solar system. In fact, when the Sun sends highly energetic particles out in to the solar system, any planets with a substantial magnetic field will interact with the particles, resulting in the emission of radio waves. Detecting this emission allows us to determine many interesting planetary properties, such as orbital parameters, habitability, plate tectonics and atmospheric compositions. Yet we have not observed any auroral activity from planets that lie outside our solar system (we have, however, detected radio auroral emission on a brown dwarf star!).

Proxima b

Figure 1: Artist’s impression showing Proxima b orbiting its red dwarf host Proxima Centauri. [ESO/M.Kornmesser]

Today’s bite investigates our nearest stellar neighbour, Proxima Centauri, to answer one very important question: can we detect auroral emissions from its exoplanet, Proxima b?

What Are We Looking For?

To answer this question, the authors first employ radiometric Bode’s law to estimate the magnitude of radio waves released during stellar wind and magnetosphere interactions. Radiometric Bode’s law, derived from observations of magnetic planets within our own solar system, indicates that the brightness of radio waves increases with size of the planetary magnetosphere. Blackett’s law then suggests the size of the magnetosphere scales with mass and rotation speed, which translates to a predicted radio power of 1013 for Proxima b. When combined with Proxima b’s estimated magnetic field strengths of 0.007 – 1 G, comparable to the 0.5 G observed at the Earth’s equator, scientists expect the frequency of these radio waves to be between 0.02 – 2.8 MHz.

radio wave brightness

Figure 2: Predicted brightness of radio waves versus emission frequency, in accordance with Bode’s law, for 106 exoplanets. The expected values for Proxima b are highlighted in the blue square, with the yellow star representing a magnetic field value of 0.3 G. The black dashed line indicates the cut-off frequency for Jupiter. [Burkhart & Loeb 2017]

Figure 2 highlights the first hurdle for observations of radio auroral emissions from Proxima b. All radiometric modelling points to radio waves being emitted at frequencies between 0.02 – 2.8 MHz for this exoplanet. However observations below 10 MHz are not possible from Earth, as these wavelengths are blocked by our atmosphere — so even at the extremes of likely magnetic field strengths, we cannot use ground-based instrumentation. A secondary issue with observing aurorae on Proxima b is that we’re now observing in a low-frequency regime that tends to be absorbed by the interstellar medium (ISM).

Modelling a Magnetosphere

So far, the authors have only estimated the brightness of auroral activity on Proxima b. With its close-in orbit creating a highly variable magnetosphere radius, we expect the variation in the observed radio waves to be rather large. To characterise this variability, authors implemented models of the wind and magnetic field around Proxima b as a function of various orbital parameters. An example of the results obtained from the simulations is shown below in Figure 3.

Figure 3: Variation in the observed radio brightness as the radius of the magnetosphere caries over Proxima b’s short 11.2 day orbit. Eccentricity = 0 and inclination = 10 degrees. [Burkhart & Loeb 2017]

Here we see how the simulated brightness of radio waves changes over one orbit. The two dips correspond to periods where the magnetospheric radius suddenly changes as the planet passes through streamer regions, located near Proxima Centauri’s equator, where stellar winds become denser. The authors also considered four different magnetic field strengths and found that a weaker magnetic field, and therefore lower emission frequency, results in brighter radio emission.

From modelling the variability in radio flux for Proxima b, the authors concluded two things:

  1. Proxima b’s radio auroral emissions vary by almost an order of magnitude over one full orbit.
  2. The amplitude of the variation depends on orbital parameters — the eccentricity and inclination of the orbit — as well as on parameters of the stellar wind and planetary magnetic field.

Can We Detect Aurorae Around Proxima b?

There are a number of issues associated with undertaking observations of Proxima b’s radio emission. As previously mentioned, the ISM is highly problematic, as electrons within the ISM are more likely to absorb photons at low frequencies due to free-free absorption. Thankfully, this shouldn’t be an issue above 0.3 MHz for nearby planetary systems, like Proxima b, as there is less ISM to contend with. We also have to overcome the 10 MHz atmospheric cut-off introduced by absorption of photons in the ionosphere. Clearly the only solution here is to make observations from space. The authors mention several interesting proposals including a radio observatory on the Moon (one example given is ROLSS) and clusters of low-cost CubeSats to form a very large telescope (think of experiments like ALMA which combined lots of smaller telescopes to form one big one, but in space).

Figure 4: Example CubeSat with hands for scale. [NASA]

The take-home message of this paper is that the brightness of radio emissions around Proxima b are substantial enough to be detected here on Earth. This is fantastic because if aurorae, caused by interactions between stellar winds and the planet’s magnetosphere, are detected on Proxima b, we will be able to further constrain the planet’s orbital inclination, eccentricity and generally gain insight into its magnetosphere. Now we just have to wait for the right instrumentation to put into space, allowing scientists to overcome the pesky 10 MHz limit imposed by our own atmosphere.

About the author, Amber Hornsby:

First year postgraduate researcher based in the Astronomy Instrumentation Group at Cardiff University. Currently I am working on detectors for future observations of the Cosmic Microwave Background. Other interests include coffee, Star Trek and pizza.

NGC 1300

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Galaxy-Scale Bars in Late-Type Sloan Digital Sky Survey Galaxies Do Not Influence the Average Accretion Rates of Supermassive Black Holes
Authors: A.D. Goulding, E. Matthaey, J.E. Greene, et al.
First Author’s Institution: Princeton University
Status: Accepted to ApJ, open access

When it comes to picking their host galaxies, active galactic nuclei (or AGN) are rather promiscuous. They reside in all types of galaxies: ellipticals, irregulars, and spirals. AGN of the same feather tend to flock together — the more luminous and radio-loud ones are found in elliptical galaxies while the lower luminosity ones are more often found in spiral galaxies. This is a manifestation of the black hole mass-host galaxy luminosity correlation, where spiral galaxies like our Milky Way tend to have less massive black holes than elliptical galaxies. Besides spiral arms, spiral galaxies sometimes also boast of having bars, if the right mood strikes. How are bars related to their AGN? Could they trigger the central black holes to light up as AGN?

Galactic bars are thought to contribute to the dynamical evolution of their host galaxies. Numerical studies show that they can funnel in gas from the outskirts to the central regions of the galaxies, triggering star formation and possibly AGN activity. It is still unclear whether bars actually help trigger AGN, as previous studies have produced conflicting results and tend to suffer from small number statistics and biased AGN diagnostics. In today’s paper, the authors bring better tools to bear on the problem, by utilizing the large wealth of information from the SDSS Galaxy Zoo citizen science project and X-ray stacking analyses.

The authors first selected a sample of ~100,000 local spiral galaxies from SDSS that have been visually classified as such in Galaxy Zoo by at least 20 people. This sample is later divided into three redshift bins that are each complete in stellar mass, i.e. there are galaxies that span the whole range of stellar masses in each redshift bin. This is to ensure the final results are representative and unbiased. They distinguished sources with and without bars in these three redshift bins using information from Galaxy Zoo, based on the fraction of votes to the question “Is there a sign of a bar feature through the center of the galaxy?” If the fraction of votes is equal or greater than 0.25, the galaxy is defined to have a bar; if the fraction of votes is less than 0.1, the galaxy is defined to be bar-less. Galaxies with fraction of votes between these two numbers are defined to be ambiguous. Figure 1 shows some examples of spiral galaxies with bars, no bars, and ambiguous bars in their sample.

Fig. 1: Sample unbarred (blue borders), ambiguously barred (yellow borders), and barred (red borders) spiral galaxies from the Galaxy Zoo project, as determined by fbar, which is the fraction of votes by citizen scientists for the presence of bars. [Goulding et al. 2017]

In contrast to optical light which is absorbed by dust, X-rays from an AGN can more easily pierce through dust obscuration. Stacking lots of X-ray observations help to reveal heavily-obscured or low-luminosity AGN. Using data from the Chandra X-ray observatory, the authors performed X-ray stacking analyses to investigate the presence of AGN in their barred, unbarred, and ambiguously barred samples. Figure 2 shows the X-ray luminosity of their samples after subtracting the contributions from star formation processes. The three types of spiral galaxies do not show obvious differences in their X-ray luminosities, suggesting that AGN are no more common in one type of galaxy than the others. For galaxies with X-ray detections (i.e. hosting AGN), the authors further investigated the distributions of their specific black hole accretion rates, which are the X-ray luminosities divided by the host galaxy stellar mass shown in Figure 3. This ratio removes the dependence on stellar mass and instead probes the dependence on the host galaxy properties. There is again no difference in the accretion rates between the barred and unbarred samples.

Fig. 2: Star-formation subtracted X-ray luminosities vs. redshift in three X-ray energy bands. As with Figure 1, blue markers refer to unbarred galaxies, yellow to ambiguously barred, and red to barred galaxies. Open markers are sources with X-ray detections while filled markers are the luminosities produced by stacking sources without X-ray detections. As a comparison, the predicted mean X-ray luminosities due to stellar processes are shown by the dotted lines. [Adapted from Goulding et al. 2017]

Fig. 3: Distributions of specific black hole accretion rates for galaxies with AGN. The different line colors again refer to the presence or absence of bars. Dashed, solid, and dotted lines refer to different cuts in the X-ray luminosity. [Goulding et al. 2017]

Well, all that is a bummer — the presence of AGN in spirals seems to be independent of the presence of bars. For those with AGN, there is also no difference in the specific accretion rates of their host galaxies on the basis of a bar existence. As stacking analyses tend to wash away short timescale events, any bar contributions to AGN activities would need to be very short-lived. This study shows that over the lifetime of the galactic bars, they do not play significant role in triggering AGN — astronomers need to turn their eyes to other means of growing black holes in spiral galaxies.

About the author, Suk Sien Tie:

I am a third year PhD student at the Department of Astronomy at The Ohio State University. I am currently working on quantitative analyses of various quasar selection methods using the Dark Energy Survey (DES) and quasar variability via microlensing.

exoplanet atmosphere

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Redox Evolution via Gravitational Differentiation on Low Mass Planets: Implications for Biosignatures, Water Loss and Habitability
Authors: R. Wordsworth, L. Schaefer, R. Fischer
First Author’s Institution: Harvard University
Status: Submitted to ApJ, open access

Looking for Life

If you’ve been tuning into astronomy news lately, you’ve probably heard about a number of cool new exoplanet discoveries, like those in the TRAPPIST-1 system, continuously rolling in from our telescopes hard at work. But no matter how, when, and where a new exoplanet is discovered, there’s always that question burning at the back of our minds: could this exoplanet have Earth-like life?

Figure 1: A screenshot from the open-universe space exploration video game called No Man’s Sky. Even with Earth-like constraints, the sky’s the limit in terms of the many living worlds we can imagine are out there. [No Man’s Sky].

This question is certainly not an easy one to answer. For an exoplanet to house life (as we understand life so far), there’s a long checklist of requirements (like those discussed here and also here) that we need the exoplanet to fulfill. For example, life as we know it survives and thrives on liquid water, so we require that the exoplanet has the ability to hold liquid water.

Today’s astrobite focuses on another important requirement: the exoplanet’s atmospheric composition. Here on Earth, for instance, we have a lot of wonderful plant-based and plant-like creatures (like trees) that produce oxygen through photosynthesis. Then other creatures here (like humans) use that oxygen to survive and thrive. So for an exoplanet to have Earth-like life, we expect it to have a buildup of oxygen.

You might imagine, then, that we should be looking for oxygen on these exoplanets, as a sign of Earth-like life — and you’d be right, mostly! But unfortunately it’s not clear that a buildup of oxygen will “always” be a sign of Earth-like life. Instead, an exoplanet could possibly accumulate oxygen from purely chemical, completely not-organic-or-life-related (aka, abiotic) processes. This means that if we find an exoplanet that has a buildup of oxygen, we need to be cautious and somehow make sure that we haven’t just discovered a false positive (i.e., a case where what we think to be true is actually very false).

Today’s authors present a nifty theoretical framework for thinking about and modeling the atmospheres of exoplanets, which can help both characterize and predict the atmospheres of the many new exoplanets we’ve observed and continue to observe. The authors did many, many other cool things in their paper, but here we will focus on understanding the backbone of their theoretical framework.

A Little Chemistry

The authors use the concepts of ‘redox’ as the main variable of their model. ‘Redox’ is an abbreviation for ‘oxidation-reduction reaction‘, which is a fancy term for a reaction involving the exchange of electrons between two chemical species. The atom, molecule, or ion gaining an electron(s) is ‘reduced’, while the atom, molecule, or ion giving up an electron(s) is ‘oxidized’. Combustion is a common example of a redox. When you burn firewood at, say, a campfire, the carbon (C) from the wood reacts with the oxygen (O2) in the air, producing carbon dioxide (CO2). In this case, the carbon gives up four electrons and is oxidized, while the oxygen collectively gains four electrons and is reduced.

If a planet has an atmosphere that is very oxidizing, that means there is, in a sense, a net demand for electrons. That means we would expect O2, which wants to take electrons, to abiotically build up in an oxidizing atmosphere, because there would be no net supply of chemical species floating around for the O2 to react with and take electrons from. So to evaluate if an exoplanet would likely abiotically build up O2, we want to check if and when its atmosphere is likely to be oxidizing — and that depends on the planet’s chemical composition.

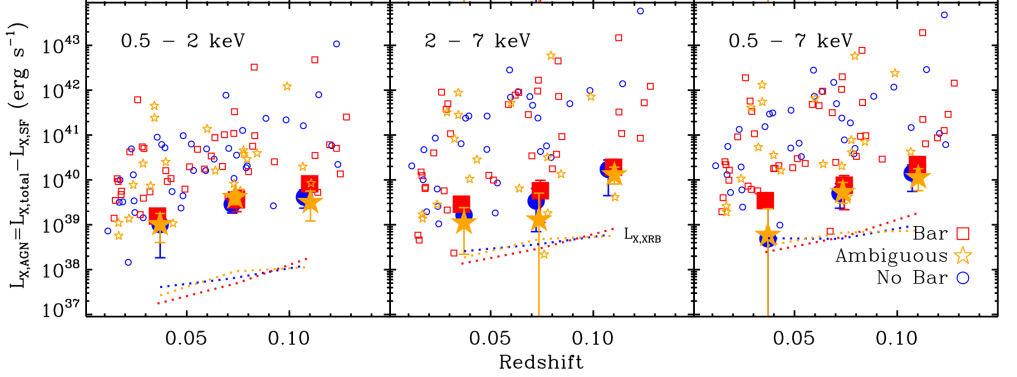
Figure 2 shows different elements from the periodic table as a function of electronegativity (which is basically how oxidizing versus reducing the elements are) plotted against atomic mass and abundance. With Earth as our example, we expect that the less massive elements like hydrogen (H), carbon (C), nitrogen (N), and oxygen (O) largely hang out in the volatile layer (which includes the atmosphere) and upwards towards space; magnesium (Mg), silicon (Si), and sulfur (S) largely get caught up in the planetary crust and mantle; and the heavy element iron (Fe) is largely trapped down in the core.

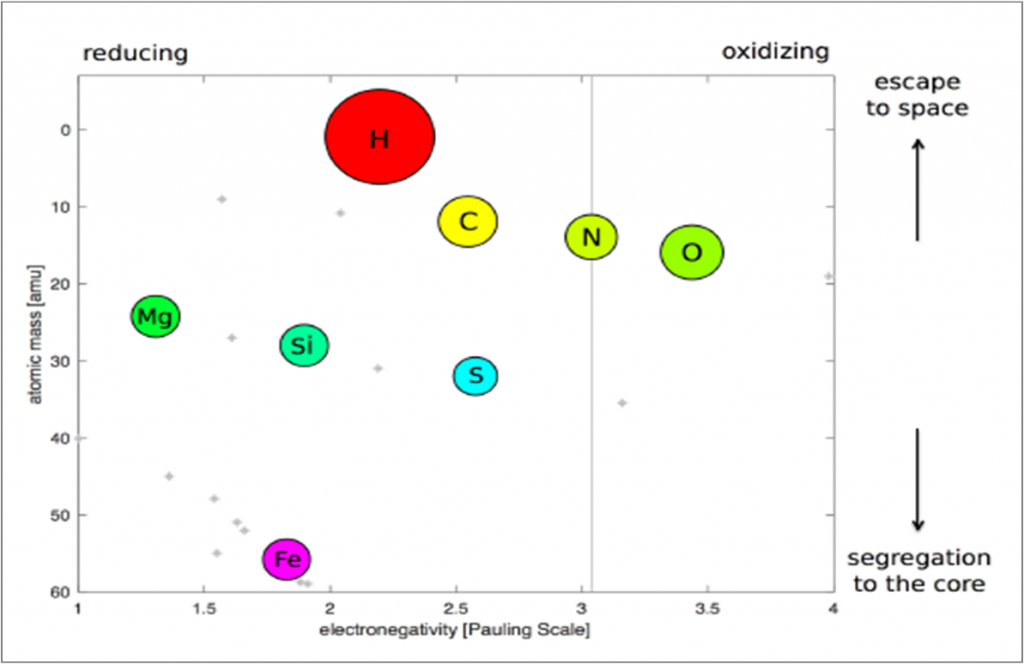
Figure 2: Electronegativity (x-axis) versus atomic mass (y-axis) for the most abundant elements in our solar system. Each circle corresponds to an element, which are labeled according to their periodic table abbreviations (H is hydrogen, for example). The sizes of the circles reflect the relative logarithmic abundances of these elements in our solar system. The faint gray asterisks in the background label elements less than 10% of the abundance of silicon (Si). Reducing elements are towards the left of the plot, while oxidizing elements are towards the right. And overall, the less massive elements rise towards space, while the more massive elements fall towards the planet’s core. [Wordsworth et al. 2017]

Studies have shown that, just like Earth, planets of around 1 to 10 Earth masses tend to split into these three major layers: volatile, mantle, and core. So to understand how oxidizing the atmosphere of a planet in this mass range is, we need to understand how these three planetary layers interact and exchange these different elements.

Putting It All Together

The authors quantify how oxidizing a planetary layer is in terms of the layer’s total oxidizing power using the following equation (Equation 1 in the paper):

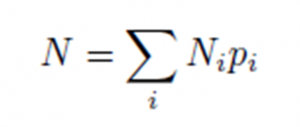
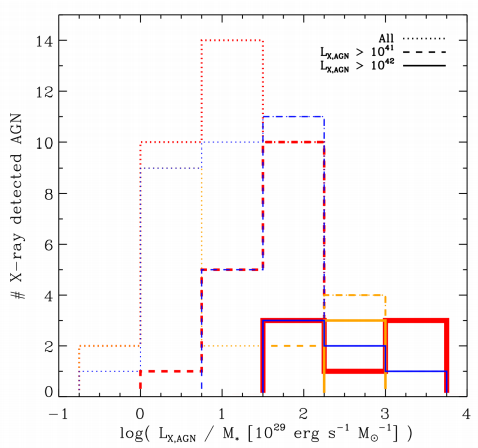
Where N is the total oxidizing power, Ni is the number of atoms of some element ‘i’ (like carbon or oxygen), and pi is the oxidizing potential of element ‘i’. An atom of oxygen, for example, takes two electrons, so it has an oxidizing potential of +2. An atom of hydrogen, on the other hand, gives away one electron, so it has a negative oxidizing potential of -1. Finally, the Σ in the equation says that we want to sum over all elements ‘i’ in the layer. The authors drew out a model of the three-layer planetary system, as shown in Figure 3, and assigned a total oxidizing power N for each layer.

Figure 3: A three-layer model for a planet of about 1 to 10 Earth masses after formation. The N at the bottom-left of each layer represents that layer’s total oxidizing power. The k terms represent the exchange between these layers over time. And the E at the top represents the escape of the less-heavy elements, especially the reducing element hydrogen, out into space. [Wordsworth et al. 2017]

To calculate how oxidizing the atmosphere is with this model, we must track the flow of elements between these three layers over time. The authors discuss a lot of the cool processes that can allow transport of material between these layers at different times in the planet’s evolution. For example, when the planet is young, its mantle layer can be partially or fully ultra-hot and molten, like an ocean of magma. During this phase, the liquidy mantle layer and the atmosphere-containing volatile layer can interact and exchange materials much more easily. But once the planet cools down and the mantle layer hardens, the interactions get more complicated. Depending on the planet’s geodynamic structure (such as any churning plate tectonics and gas-spewing volcanoes), the mantle layer can shift around and still allow material to flow between these planetary layers. And over time the much-less massive elements, especially the reducing element of hydrogen, can leak out of a planet’s atmosphere and escape to space (that’s the ‘E’ term in Figure 2), also changing how oxidizing the atmosphere is.

Once we have a good handle on how these conditions and processes play out on other planets — such as answers to the tough question of how geodynamics evolve on planets other than Earth — we can apply this framework to dig out any planetary scenarios where compounds like O2 could build up abiotically over time. And for those seemingly habitable exoplanets that appear very unlikely to abiotically build up O2, we can be cautiously optimistic that any oxygen buildup we do see might come from a biotic source — exactly what we’ve been searching for all along.

About the author, Jamila Pegues:

Hi there! I’m a 2nd-year grad student at Harvard. I focus on the evolution of protoplanetary disks and extra-solar systems. I like using chemical/structural modeling and theory to explain what we see in observations. I’m also interested in artificial intelligence; I like trying to model processes of decision-making and utility with equations and algorithms. Outside of research, I enjoy running, cooking, reading stuff, and playing board/video games with friends. Fun Fact: I write trashy sci-fi novels! Stay tuned — maybe I’ll actually publish one someday!

PSR J1023+0038

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we occasionally repost astrobites content here at AAS Nova. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Simultaneous Chandra and VLA Observations of the Transitional Millisecond Pulsar PSR J1023+0038: Anti-correlated X-ray and Radio Variability
Authors: Slavko Bogdanov, Adam T. Deller, James C. A. Miller-Jones, et al.
First Author’s Institution: Columbia University
Status: Submitted to ApJ, open access

What’s more interesting than a rapidly spinning neutron star that emits electromagnetic radiation parallel to its magnetic poles? One that doesn’t exactly behave as expected, of course. One such weirdly acting pulsar, PSR J1023+0038, is a transitional millisecond pulsar (tMSP) — which is fancy speak for a pulsar with a millisecond rotational period that switches between radio and X-ray emission on a several-year timescale. The fact that this pulsar emits in both X-ray and radio on these longer timescales isn’t what piques the interest of astronomers, however, in the case of the study in this astrobite.

Weird Pulsar Behavior

Figure 1: Radio emissions (black) and x-ray emissions (blue) recorded by the VLA and Chandra respectively over time. This shows that when radio emissions drop off, X-ray emissions pick up.

Pulsars can typically fall into one of the following categories: radio pulsars are powered by exchanging rotational energy from the spinning neutron star into emitting radiation. This means that their rotation slows and their pulse length increases. Meanwhile, X-ray pulsars are accretion powered, meaning they turn heated infalling matter into X-ray emission. What distinguishes PSR J1023+0038 from the background of pulsars that switch between accretion-powered X-ray and rotation-powered radio pulsars is that it has a simultaneous anti-correlated X-ray and radio emission. The authors looked at about 5 hours of overlapping and concurrent observations from the Chandra X-ray Observatory and the Very Large Array (VLA) to try and understand this weird relationship between the X-ray and radio emissions. This is very clearly shown in Fig. 1 where we can see a tiny sample of time of overlapping X-ray and radio flux measurements. The anti-correlation is quite strong, meaning that when the X-ray emissions are weakest, the radio emission is strongest.

Figure 2: (Top) Chandra x-ray observations over a period of 5 hours.The 3 x-ray modes can be seen as the one large peak (~12.7 hrs), the low (minima), and high (steady maxima).    (Middle) The simultaneous observation as seen from the VLA. (Bottom) The overlapped top and middle observations show the anti-correlation between x-ray and radio emissions.

But wait…there’s more! When we zoom out on the flux/time series observations (Fig. 2) we can not only see that the anti-correlation is persistent, but we can also see that the X-ray emission has at least 3 unique modes of operation. The authors classify these X-ray emission modes as (1) sporadic flaring (~12.7 hrs in Fig. 2), (2) high, and (3) low modes. The difference between high and low in this context is the magnitude of the luminosity.

Trying to Explain Away the Strangeness

This complex and weird behavior unfortunately does not come with an easy or readily available answer. What we know about pulsars and how we can model pulsar accretion doesn’t shed any new light on the situation. The authors do suggest that the switching between high and low modes might occur due to a changing unstable magnetosphere. They also propose that the increase in radio emission can be explained by an outflow of plasma that emits synchrotron radiation as it travels. Additionally, when comparing PSR J1023+0038 to a low-mass X-ray-emitting binary black hole (BH LMXB) (Fig. 3), we can see that the low mode of this tMSP falls into the binary BH region. This is unusual because there is a pretty clear separation between the X-ray/radio luminosity relationship of neutron stars and BHs. Knowing this now, it may call into question whether some BH LMXBs could have been misidentified.

Figure 3: PSR J1023+0038 low (red diamond) and high (red pentagon) X-ray modes closely follow the accreting low-mass X-ray binary black hole systems, making it indistinguishable in the low mode.

Now you may be asking, “so what did we actually discover?”, which is a completely valid question. Well for one, we learned that there in fact do exist strange and unique pulsars that exhibit odd behavior. But the more exciting result is that we may not have a great understanding of pulsars in general. This is exciting because it can spur new astrophysical theories and models; ones that can more generally explain even the weirdest behaviors. Like most of astronomy (and science in general) however, before we can fully claim any specific mechanism for causing the anti-correlated X-ray and radio emissions and the switching between emission modes, we’ll probably need more observations.

About the author, Joshua Kerrigan:

I’m a 3rd Year PhD student at Brown University studying the early universe through the 21cm neutral hydrogen emission. I do this by using radio interferometer arrays such as the Precision Array for Probing the Epoch of Reionization (PAPER) and the Hydrogen Epoch of Reionization Array (HERA).

Hyades cluster

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: K2-NnnA b: A Binary System In The Hyades Cluster Hosting A Neptune-Sized Planet
Authors: David R. Ciardi, Ian J. M. Crossfield, Adina D. Feinstein et al.
First Author’s Institution: Caltech/IPAC-NASA Exoplanet Science Institute
Status: Submitted to AJ, open access

The authors of today’s paper report the discovery of a Neptune-sized planet in the nearby Hyades Cluster orbiting within a binary star system. A binary consists of two stars mutually orbiting their center of gravity; the larger star is called the primary and the smaller is the secondary. In a close binary where the stars are separated only by a short distance, each of the two stars affects the evolution and size of the other.

The binary system in today’s paper is referred to as EPIC 247589423 (also called LP 358-348), but the primary and secondary stars within this system are called K2-NnnA and K2-NnnB, respectively. Since the planet discovered in this system orbits just the primary star, the authors of this paper refer to it as K2-NnnA b: exoplanets are generally named after their host star and then have another letter added to the end. However, this planet does not yet officially have a name, so there is hope that it will be called something that rolls off the tongue a bit more easily.

Significance to Planetary Formation and Evolution

Planets in binaries form under the influence of two stars — an extreme environment for planet formation, particularly if the two stars are close. The system EPIC 247589423 has a very close separation of 40 AU, which is roughly the distance from Pluto to the Sun. Using our solar system as an example, if we replaced Pluto with a star, it is easy to see that the rotation and generally everything about the planets would be drastically affected.

Studying binary-orbiting planets like K2-NnnA b allows us to test the robustness of planet formation, since the planets have to survive in an extreme environment for a long time. These systems also let us test how often planets are retained by their host star rather than being destroyed by the changing gravitational field. What’s more, by finding and studying planets in star clusters, we may begin to understand how planetary systems form and evolve and find out the timescale for such events.

K2 and Follow-up Observations

K2, or as I like to call it, Zombie Kepler, is the current mission for the Kepler Space Telescope. The Kepler Space Telescope was launched in 2009 with the mission of finding exoplanets through transit photometry. The method of transit photometry means recording the brightness of the star for an extended period of time, then looking for any small periodic ‘dips’ in the brightness that occur when an exoplanet eclipses a small part of the star. This data is generally referred to as a light curve, since there is a rounded dip where the exoplanet eclipses the star. After a mechanical mishap in 2013 with the Kepler Space Telescope, the scientists working on this mission still found a way to use the telescope to look for transiting exoplanets. Instead of observing one single part of the sky for an extended period of time as before, K2 now observes smaller patches of the sky for shorter amounts of time. Figure 1 shows several stages of the light curve analysis from K2.

Figure 1: This shows the light curve of EPIC 247589423 in various stages of analysis from K2. The topmost panel shows the light curve with the telescope rotation removed. The second panel shows the binned version of the top panel. The third shows the data with the stellar variability removed, and the lowest panel shows the folded and binned result for the planet transit. [Ciardi et al. 2017]

Scientists discovered the short-period exoplanet in the EPIC 247589423 system by examining the star’s K2-obtained light curve. Since K2-NnnA b orbits a binary system, the researchers had to first remove the stellar variability inherent in the data. The two stars within the binary already eclipse each other and cause dips in the light curve, which can make it difficult to find a much smaller dip from the planet. After removing the eclipse variability, they found the planet and solved for its period and radius.

After the initial discovery with K2, the researchers followed up their detection with new observations and archival data to confirm the planet’s presence. They used the archival data from 1950 Palomar Observatory Sky Survey to rule out any other object that could be causing the dip in the light curve — for example, another eclipsing binary behind the system, which could create variation in the light curve that could be mistaken for a planet — and then made additional observations of the system using the Keck I telescope.

K2-nnnA b: Planet Properties

The EPIC 247589423 binary system is located in the nearby Hyades cluster — a cluster roughly 750 Myr old and the nearest star cluster to the Sun. The two stars are separated by about 40 AU. The planet orbits the star K2-NnnA with a period of 17.3 days, and its transit lasts roughly 3 hours.

K2-NnnA b is one of the first Neptune-sized planets that has been observed orbiting in a binary system within an open cluster. The discovery of this planet can provide us with a better understanding of the planet population in stellar clusters and allow us to place more limits on planetary formation and evolution.

The authors of this paper say planets discovered in nearby star clusters ‘provide snapshots in time and represent the first steps in mapping out [planetary]evolution,’ and I wholeheartedly agree. The discovery of K2-NnnA b brings with it new understanding of planet formation in star clusters and in binary systems. The possibilities of planet formation and evolution are certainly not limitless, and with more and more discoveries like K2-NnnA b, we can hopefully find the extremes of planetary systems.

About the author, Mara Zimmerman:

Mara is working on her PhD in astronomy at the University of Wyoming. She has done research with Heartbeat binary stars and currently works on modeling debris disks.

1 … 30 31 32 33 34 … 38