Astrobites RSS

Titan

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Compositional Similarities and Distinctions Between Titan’s Evaporitic Terrains
Authors: S.M. MacKenzie and Jason W. Barnes
First Author’s Institution: University of Idaho
Status: Published in ApJ, open access

Titan, Saturn’s largest moon, is the only solar system object other than the Earth to have a thick atmosphere and standing surface liquid. When the Cassini spacecraft began observing Titan, it even discovered lakes and seas dotting the northern hemisphere. Don’t fire up your rocket just yet, though — because Titan is so cold, the lakes and seas are filled with liquid methane and ethane rather than water.

Cassini

Artist’s illustration of the Cassini mission at Saturn. [NASA]

Titan’s thick, methane-rich atmosphere makes it difficult to observe the surface at visible wavelengths. Luckily, there are several windows in the near-infrared through which light can pass and reveal the surface. Seven of these windows overlap with the wavelength range covered by Cassini’s Visual and Infrared Mapping Spectrometer (VIMS). By looking at how the brightness of the surface changes with wavelength, we can learn about the composition of the surface material. The cover photo above depicts a three-color map of Titan’s surface made with VIMS. The pinkish regions show where the surface reflects strongly at 5 microns.

The 5-micron-bright regions are found circling lakes in the northern hemisphere, in dry lake beds in both hemispheres, and in the desert-like equatorial regions. The bright rings around the lakes are believed to be evaporites—solid material left behind after the liquid in which it was dissolved evaporates. This explains the presence of the bright material surrounding the lakes and the dry lake beds, but what about the desert? Linking 5-micron-bright regions in what is today a desert to the bright rings around the lakes could provide evidence that the equatorial regions of Titan were once covered with liquid.

In this paper, the authors searched for a compositional link between the bright regions in the desert and the evaporites around the lakes and seas. They used an absorption feature at 4.92 microns in order to investigate whether or not the 5-micron-bright material in each of these regions is the same. The 4.92-micron absorption feature has been observed previously in the desert region, but no one has been able to definitively say what compound causes it. Because of this, finding the same feature in the desert and around the lakes can indicate that the regions are geologically similar, but can’t yet tell us about the chemical makeup of the material.

VIMS data

A non-projected version of the VIMS map of Titan shown above. [JPL/NASA/Univ. of Arizona/CNRS/LPGNantes]

The authors used Principal Component Analysis (PCA) to isolate the weak 4.92-micron absorption feature. PCA is a mathematical method that separates the individual components that make up an observed signal. In this case, the main contributors to the signal (i.e. the “principal components”) could be changes in the surface reflectivity, instrumental noise, or compositional variations. Once the components have been separated, the unwanted contributors can be removed. As a result, PCA can be used to isolate a signal that is much smaller than the background noise. (PCA is also used in the direct detection of exoplanets and is described in more detail here.) After applying PCA, the authors observed the 4.92-micron absorption feature in both the desert and around the lakes, strengthening the hypothesis that the desert once had liquid. However, they also found that not all of the lake regions had the absorption feature, and some of the regions that did have it didn’t have it in every observation. They suggested that material with a crystalline structure that reflects light more strongly at some angles or transient effects like methane rain could cause the absorption feature to appear intermittently.

What causes some lake regions to have the absorption feature while others don’t? The authors suggested that the material that causes the 4.92-micron absorption feature could be just one of several solids that are left behind as the lakes evaporate away. Whether or not a lake rim has the absorption feature could be a function of how far the evaporation has progressed. As evaporation proceeds, materials that are more soluble precipitate out in sequence. We could see a 5-micron-bright evaporite ring without the absorption feature if the lake hasn’t evaporated enough for the material causing the absorption to precipitate out. The authors even have a suggestion for why this might happen to some lakes in the northern hemisphere but not others—lakes closest to the north pole might experience more methane rainfall than more southern lagoons, periodically halting the evaporation before the absorbing material can crystallize.

Although the authors posit many explanations for the mysterious behavior of the 4.92-micron absorption feature, they can’t yet settle on one cause. It’s not surprising that Titan, an inhospitable but strangely familiar world with complex geology and weather systems, presents a challenge to astronomers. In the future, by modeling how Titan’s climate changes over time, we can hope to learn more about what causes the distribution of evaporites on Titan’s surface.

About the author, Kerrin Hensley:

I am a second year graduate student at Boston University, where I study the upper atmospheres and ionospheres of Venus and Mars. I’m especially interested in how the ionospheres of these planets change as the Sun proceeds through its solar activity cycle and what this can tell us about the ionospheres of planets around other stars. Outside of grad school, you can find me rock climbing, drawing, or exploring Boston.

hot Jupiter atmosphere

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Atmospheric circulation of hot Jupiters: dayside–nightside temperature differences
Authors: Thaddeus D. Komacek and Adam P. Showman
First Author’s Institution: University of Arizona
Status: Published in ApJ, open access

There is an old sci-fi movie “The Chronicles of Riddick” that’s set on a bizarre planet. One scene I still remember depicted the dawn on the planet. As the sun was rising (yes, somehow not tidally-locked…), the frozen surface from the night suddenly became so boiling hot that it burned anything into ash within seconds. Fortunately, on Earth, the day–night temperature difference is much milder — it’s usually less than 30°C, because the atmosphere mitigates the temperature and the Earth rotates quickly (like making evenly grilled chicken by turning it).

Hot Jupiters, on the other hand, are extreme worlds. They orbit very close to their host stars (< 0.1 AU) and are locked by the tidal force into synchronous rotation, with the same side always facing their stars. This makes for interesting atmospheric dynamics. In today’s astrobite, we take a look at these exotic worlds. The authors examined what essentially controls the day–night temperature differences and compare their theory to numerical simulations (so-called general circulation models or GCM).

The Hotter It Is, the Faster It Cools

Figure 1. Normalized day–night brightness temperature difference vs. equilibrium temperature from observation of transiting hot Jupiters.

Figure 1 summarizes the observed day–night temperature difference vs. the equilibrium temperature of several most-studied hot Jupiters. The value shown on the y-axis is the normalized day–night temperature difference. The equilibrium temperature shown on the x-axis essentially tells us how close the planets are to their stars. There is clearly a trend, and the reasoning can be understood via the Stefan–Boltzmann law: the hotter (more strongly irradiated) the planet, the faster it cools (radiates). The winds cannot carry hot gas to the planet’s nightside if the heat is radiated into space too quickly. This would lead to a larger day–night temperature difference. On the other hand, if the atmospheric circulation acts efficiently enough, the winds can smear out the temperature variations by redistributing heat across the planet. Another way to look at it is that hotter planets have shorter radiative timescales. Astrophysicists like to think in terms of “timescales” while dealing with different competing processes. The process with a shorter timescale occurs faster, and it therefore dominates over the others. The authors developed a simple analytical theory that shows the dependence of day–night temperature difference, as a function of several factors: day–night equilibrium temperature difference, the timescale of radiation, and the timescale of drag. These factors, together with planetary parameters, are the key input of GCM simulation and allow a direct comparison.

Scale Analysis

Scale analysis is a powerful tool for understanding which mechanisms play important roles. The authors use scale analysis to simplify the problem and construct the theory. For example, by looking at the continuity equation, we can readily understand that  U/L ~ W/H; i.e., the ratio of the horizontal wind speed (U) to the vertical wind speed (W) is about equal to that of the planet radius (L) to the pressure scale height (H). For a typical hot Jupiter, this ratio is ~100. In other words, the observable atmosphere is thin and the horizontal flow is much faster than the vertical motion.

Pen & Paper vs. Computer

Applying scale analysis to the set of primitive equations describing the atmosphere, the authors worked out analytical expressions for various quantities, like wind speed and day–night temperature difference. Before comparing with the GCM results, let’s briefly follow their analysis based on the governing law of the gas movement — the conservation of momentum. The gas motion has four contributing factors: gas flowing in or out (advection), planet’s rotation (Coriolis force), drag force (friction), and how the pressure changes spatially (pressure gradient). The pressure gradient is the driving force of the circulation, which has to be balanced by the others. Depending on whether drag, Coriolis force, or advection dominates, the atmosphere may be in different regimes and behave differently. When rotation is important compared to advection, the pressure gradient is balanced by rotation (weak-drag) or by drag force (strong-drag), but when rotation is negligible compared to advection (slow rotation or near the equator), the pressure gradient is balanced by advection (weak-drag) or by drag force (strong-drag).

Figure 2. Overall day–night temperature difference (colors) as a function of radiative timescale and drag timescale, showing a comparison of the GCM results (left) and theory (right). The black line in the right panels indicates the transition from drag dominance to rotation dominance.

The theoretical prediction is compared to GCM results in Figure 2, showing the day–night difference for various values of drag timescales and radiative timescales for the rotation-important regime. Firstly, the day–night difference decreases as radiative timescale increases, in accordance with the trend observed. Secondly, the day–night difference has no dependence on the drag timescale until the drag timescale is shorter than ~105 s (the black line in the righthand panels). This indicates the transition as discussed above: the weak-drag regime lies above the black line and the strong-drag regime under it. Lastly, a term that corresponds to the timescale of wave propagation appears in the analytical solutions in all regimes. These waves are similar to the waves in the tropics on Earth, which are strongly connected to the climate. The relative value of the wave timescale essentially controls the day–night temperature difference. It implies the wave propagation is crucial to mediate the day–night temperature difference (read more about this here).

In addition, the circulation and temperature structures in the GCM are displayed in Figure 3. Again, the radiative timescale predominantly determines the day–night temperature difference, until the drag becomes strong enough and the equatorial jet vanishes, exhibiting a symmetric circulation pattern.   

Figure 3: How temperature (colors) and wind (vectors) in GCM simulations vary with drag timescales (the y direction) and radiative timescales (the x direction).

This simple scaling theory helps us to understand the leading role of the dynamics and tells us a useful story about the physics behind it. It is always nice to see successful pen-and-paper solutions that help decipher the intricate results of computer simulations!

About the author, Shang-Min Tsai:

I am a 3rd year PhD student at the University of Bern and part of the Exoplanets & Exoclimes Group led by Prof. Kevin Heng. We are developing various open-source tools to study exoplanets. I work on modelling of atmospheric chemistry and dynamics. When I am not coding or debugging, I enjoy basketball and playing board games.

M67

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Galactic Doppelganger: The chemical similarity among field stars and among stars with a common birth origin
Authors: M. Ness, H-W. Rix, David W. Hogg et al.
First Author’s Institution: Max Planck Institute for Astronomy, Germany
Status: Submitted to ApJ, open access

Stars are not born alone. Stellar formation occurs inside giant molecular clouds, which collapse when the internal gas pressure cannot overcome gravity. This is known as Jeans instability. The increase in density caused by the collapse leads to a fragmentation of the cloud, forming thus not only one, but many stars from the same original material. Consequently stars that are born together have the same initial composition. In addiction, these sibling stars are subjected to each others’ gravitational pull, so the formation process usually results in a stellar cluster rather than isolated stars.

Some stars can be ejected from the cluster in many-body interactions. Moreover, most clusters don’t survive long, since they are disrupted due to gravitational interaction with the surroundings. This implies that siblings are not always found together. They still preserve, though, one common birthmark: their similar chemical composition. Thus one can in principle identify population members by comparing stellar abundances. This is known as chemical tagging, and it is widely used to characterize the components of the Milky Way and constrain its assembly history (see this bite and this bite).

However, there are a few considerations to take into account. First, because of differences in the evolutionary process, some spread in abundances is expected among sibling stars. Second, there’s always the chance that two stars that are not born together have the same current abundances by pure chance — so-called doppelgangers. These two difficulties were usually ignored in chemical tagging, since no estimate of their magnitude existed. Today’s paper aims to put an end to this limitation, using precise abundance determinations to estimate intra-cluster dispersion and doppelganger rates in the Milky Way’s thin disk.

Data and abundance estimates

To obtain a large sample of stars and achieve high-precision abundance measurements, the authors used data from APOGEE, a project that is part of the Sloan Digital Sky Survey. APOGEE takes spectra of mainly giant stars and allows abundance estimates for tens of chemical elements. APOGEE’s pipeline estimates each star’s physical parameters, effective temperature (Teff) and surface gravity (log(g)), and also element abundances. The authors’ first step was to correct the abundances for systematic uncertainties (such as those arising from dependence on stellar physical parameters) with a training sample containing thousands of field stars.

These corrections were applied to test data, consisting of spectra of about a hundred stars in seven well-studied open clusters. Fig. 1 shows the estimated abundances for Messier 67 (shown at the top of the page) as an example. As expected, there isn’t much spread in abundances for the stars that are part of the cluster. Using the same method, abundances were estimated for other 90 open clusters. The obtained uncertainties were typically 20% – 50% lower than obtained in other determinations.

Figure 1: Abundance estimate for 20 elements in M67 stars, coloured by effective temperature. The grey points are the training data. The values at the top of each subplot are mean and standard deviation of the estimates. All elements are measures with respect to Fe, except [Fe/H].

Figure 1: Abundance estimate for 20 elements in M67 stars, coloured by effective temperature. The grey points are the training data. The values at the top of each subplot are mean and standard deviation of the estimates. All elements are measures with respect to Fe, except [Fe/H]. [Ness et al. 2017]

Siblings or doppelgangers?

The results point out that the typical dispersion of abundances among sibling stars is comparable to the measurement uncertainties. This implies that clusters are unsurprisingly nearly homogeneous in their abundances. So there is no need to worry about intrinsic abundance dispersion in chemical tagging analysis, at least in the case of large-scale surveys.

Knowing that this dispersion is negligible for the APOGEE’s uncertainties, the authors next estimated the similarity between stars in the same cluster, and between stars in the field. Do stars within a cluster always look alike, in terms of abundance? Do stars in the field always look different? The answer to both these questions is no. The authors showed that by estimating the distribution of abundance differences, through computation of the chi-square for the measured abundances for each pair of stars, both intra-cluster and in the field. This was first done considering stars with similar Teff and log(g), and then also pairs with similar [Fe/H], a measure of metallicity.

As can be seen in Fig. 2, the distributions for intra-cluster pairs and field pairs are very different. The former has a median very close to the number of degrees of freedom, as is expected for a chi-square distribution when a good fit is obtained, confirming that most siblings are a good fit to one another, and thus very similar. There exists, however, some spread, indicating that siblings can be significantly different. The distribution for the field pairs, on the other hand, has a much larger spread and much larger values. That reflects the fact that field stars are usually not similar. However, about 1% of field pairs are as similar as siblings. The estimated probability that two stars chosen at random were born together is, according to the authors, much smaller than that (about 0.003%). Thus a significant fraction of these pairs is not in fact siblings, but only doppelgangers: very similar, but unrelated.

Figure 2: Top plot shows the chi-square distribution of abundance differences for pairs with similar Teff and log(g). The black histogram represents intra-cluster pairs, and the red-dashed shows field pairs. The distributions are very different, but not disjoint: some field pairs are as similar as siblings. Bottom panel shows analogous estimates, but the [Fe/H] is set to the similar value of the clusters M67 and NGC6819. Their intra-cluster distributions are shown in the blue dash-dot histogram for comparison. Even for similar metallicity, most field stars are distinguishable, but there's still a considerable number of doppelgangers.

Figure 2: Top panel shows the chi-square distribution of abundance differences for pairs with similar Teff and log(g). The black histogram represents intra-cluster pairs, and the red-dashed shows field pairs. The distributions are very different, but not disjoint: some field pairs are as similar as siblings. In the bottom panel pairs have also similar [Fe/H], close to the value of the clusters M67 and NGC6819. Their intra-cluster distributions are shown in the blue dash-dot histogram for comparison. Even for similar metallicity, most field stars are distinguishable, but there’s still a considerable number of doppelgangers. [Ness et al. 2017]

Implications for chemical tagging

Chemical tagging alone shouldn’t be used to characterize a population; certainly not in the Milky Way disk, even with the high data quality achieved by the authors. The relatively high doppelganger rate found by the authors, combined with the fact that siblings are not always chemically alike, makes chemical tagging uncertain. Other parameters, such as velocity information, should be combined with the abundance analysis. Keep that in mind!

About the author, Ingrid Pelisoli:

I am a second year PhD student at Universidade Federal do Rio Grande do Sul, in Brazil. I study white dwarf stars and (try to) use what we learn about them to understand more about the structure and evolution of our Galaxy. When I am not sciencing, I like to binge-watch sci-fi and fantasy series, eat pizza, and drink beer.

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Modeling the Historical Flux of Planetary Impactors
Authors: David Nesvorny, Fernando Roig, William Bottke
First Author’s Institution: Southwest Research Institute
Status: Accepted to AJ, open access

Asteroids have a bad reputation. They may have wiped out the dinosaurs, and they have threatened the survival of humanity in many terrible movies.

Until today’s featured paper, asteroids were also blamed for the Late Heavy Bombardment (LHB, for short) — a period of time in the early solar system when the Moon, the Earth, and the other rocky planets were hit with an unusually high number of impactors from a variety of material in space. It lasted from the Sun’s formation 4.6 billion years (Gyr) ago until the impact that created the Orientale crater on the Moon 800 million years later (3.8 Gyr ago). Planetary scientists working on the Apollo missions in the 1960s first hypothesized the LHB when astronauts brought back impact melt rocks from various craters that unexpectedly all dated back to this time. The idea was later extended to include the rest of the inner solar system when planetary scientists found similar cratering histories on each of the rocky planets. These LHB-era impacts are believed to have been caused by some combination of asteroids (from the asteroid belt), comets (from the Kuiper Belt and beyond), and leftover material from the formation of the inner rocky planets. However, it is still not clear which of these three sources was the main culprit.

To address one part of this issue, Nesvorny et al. — the authors of today’s paper — ask: Were there enough impacting asteroids to account for all of the craters on the Moon from the Late Heavy Bombardment?

How Did Asteroids End Up Impacting the Moon?

The leading idea for the source of asteroid impactors during the LHB is that they did not come from the main asteroid belt that exists today. Instead, they originated in what used to be the inner part of the belt — called the “E-belt” — that spanned from 1.7 to 2.1 AU, but became extinct (“E” is for Extinct!) after interacting with the planets in our solar system before they settled into their current orbits.

During this time period, the planets were not located where you think of them today. In the Nice model, Jupiter started out at 5.35 AU (compared to 5.2 AU today) and Saturn at 8.40 AU (compared to 9.5 AU today). Then, as Jupiter migrated inward and Saturn moved outward — both by about 0.04 AU — they moved from almost being in resonance to exactly in resonance. At this point, Saturn began to orbit around the Sun two times for every one time that Jupiter completed an orbit. This perfect alignment created complete chaos! Jupiter and Saturn quickly migrated towards their current locations, and in the process, they flung Uranus and Neptune much further away from the Sun, while also ejecting asteroids out of the asteroid belt. Many of these asteroids fled for the inner solar system, where they could then impact the Moon, the Earth, and the other inner planets.

Nice model

Figure 1. Nice Model: Evolution of the early solar system. When Jupiter (J) and Saturn (S) reached a 2:1 resonance (dotted line), they ejected asteroids out of the asteroid belt and pushed Uranus and Neptune away. There is a decent amount of evidence that something like this occurred (albeit, there are competing models), although it is unknown whether planet migration during the Nice model is directly responsible for the LHB. [Adapted from Rivkin et al. 2010]

Counting Asteroid Impacts in the Nice Model

Nesvorny et al. test whether asteroids could be responsible for the LHB by simulating the orbital evolution of a full belt of asteroids that stretches from 1.7 to 3.3 AU with a wide range of low eccentricities (0.0 to 0.4) and low inclinations (0 to 20 degrees). Concurrently, they also integrate the orbits of all of the planets (except Mercury to save computation time) according to how they evolve in the Nice model. They run this simulation from not too long before Jupiter and Saturn fall into resonance to the present day.

At the end of the simulation, they calibrate the fraction of asteroids that impact the Moon into an actual number of impacts to expect in the real solar system by comparing the fraction of surviving asteroids in the entire belt (in the simulation) to the number of asteroids in the real asteroid belt. Better yet, the authors count only the real asteroids above a certain size (e.g. 10 km, and 130 km) to figure out whether there are enough large impactors in the simulation to account for all of the large craters on the actual Moon.

As Figure 2 shows, Nesvorny et al. find that there are not enough large asteroids to explain the number of known impact craters on the Moon. Thus, asteroids from the asteroid belt could not have been responsible for the majority of the impacts during the LHB.

Number of impacts

Figure 2. Total number of impacts on the Moon above a given size (left: 50 km, 130km; right: 10 km, 20 km) for three simulations. Left: The Imbrium crater was created by a 130-km impactor, but not even one asteroid that size hit the Moon throughout its history in the simulations. Right: There are 200 craters with a 150-km diameter or more on the Moon, each of which was created by a 10-km impactor. However, fewer than 20 asteroids that size hit the Moon. [Nesvorny et al. 2017]

Other Ideas

Recent work has also explored whether comets could explain the LHB-era impacts on the Moon, but found that the rocks returned from the Apollo missions do not have the right chemical make-up — specifically, oxygen isotope ratios — to match up with comets. This leaves leftover material from when the Earth, Mars, and other inner planets formed as the most likely remaining explanation for the impacts during the Late Heavy Bombardment.

The lead author, David Nesvorny, mentions that he has submitted another paper (with Alessandro Morbidelli and several other planetary scientists) that validates the rocky planet leftovers as the main cause of the Late Heavy Bombardment. Hopefully when that paper is published, I can write another Astrobite that more conclusively finishes this important part of the story.

About the author, Michael Hammer:

I am a 2nd-year graduate student at the University of Arizona, where I am working with Kaitlin Kratter on studying planetary dynamics and planet-disk interactions through numerical simulations. I am from Queens, NYC.

Tibet Air Shower Array

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Northern sky Galactic Cosmic Ray anisotropy between 10-1000 TeV with the Tibet Air Shower Array
Authors: The Tibet AS Collaboration
First Author’s Institution: Yale University
Status: Accepted to ApJ, open access

One of the big unsolved mysteries in particle astrophysics is the origin and acceleration mechanisms of cosmic rays, or charged particles that are constantly bombarding the Earth. It is impossible to tell which astronomical source is the origin of any one particular cosmic ray. Because cosmic rays are charged particles, they are deflected on their way to Earth by our galactic magnetic field. Due to this effect, one might expect cosmic rays to arrive isotropically (in equal numbers in every direction), with slight anisotropy because of diffusion effects.  However, that is not what is observed. Instead, there is a large-scale anisotropy with an energy-dependent amplitude. This causes problems for traditional diffusion models. Studying this anisotropy is important in learning more about cosmic rays.

Today’s bite uses roughly five years of data from the Tibet Air Shower experiment, located at 4300 meters above sea level in Tibet, to provide an update in the study of the cosmic-ray anisotropy. The area of the sky they looked at is slightly larger than in their previous papers, and when combined with data from the IceCube experiment in Antarctica, allows us to have a complete picture of the entire sky in the energy range of a few hundred TeV.

New Results

new results

Left: The anisotropy, as seen in 5 different energy bins. From top to bottom, the median energy is 15, 50, 100, 300 and 1000 TeV. Right: The 1D projection of the plot on the left. The blue curve is the first harmonic fit to the data. Note how the phase changes with energy. [Amenomori et al. 2017]

Maps were binned according to energy and analyzed. A summary of results can be found below.

  • In the map corresponding to a median energy of ~300 TeV, there are two regions that can be seen by eye: one area where there is an excess of cosmic rays, and another area where there is a deficit. Only the excess is statistically significant after taking trials into account. (For a description of what “trials” are in a statistical context, check out this page about the “look-elsewhere effect“). This result is consistent with what IceCube sees in a similar energy range. The authors looked to see if this anisotropy is due to the Compton-Getting effect (i.e. if it was caused by the motion of our solar system around the center of our Galaxy). The Compton-Getting effect predicts that we would see more cosmic rays coming from the direction that the Earth is moving toward, with a deficit coming from the other direction. They concluded that this particular excess is not related to the Compton-Getting effect.
  • The anisotropy appears to be energy dependent. The maps in the 15–50 TeV range have completely different features than the maps at a few hundred TeV/1 PeV. (See the figure above for an illustration)
  • These effects are not related to seasonal variations in the performance of the detector (atmospheric effects can affect experiments such as the Tibet Air Shower array, but it was shown to be negligible in this case).

So what are the implications of this? Well, for one, studies like these give us some hints as to the origins of cosmic rays. For example, at a few hundred TeV, the most significant excess is coming from the direction of the Galactic center. However, the observed energy dependence shows that there is still a lot to learn about how cosmic rays diffuse. Changes in the anisotropy with energy may imply that the cosmic ray propagation parameters are evolving.

About the author, Kelly Malone:

I am a fourth year physics graduate student at Penn State University studying gamma-ray astrophysics. I previously received bachelor’s degrees in physics and astronomy from UMass Amherst in 2013.

elliptical galaxy

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we repost astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Morphology and the Color-Mass Diagram as Clues to Galaxy Evolution at z~1
Authors: Meredith C. Powell, C. Megan Urry, Carolin N. Cardamone, et al.
First Author’s Institution: Yale University
Status: Published in ApJ, open access

Introduction

In astronomy, the relationship between the color and the brightness of objects has been used as a basic classification tool for decades. For stars, color is a measure of temperature; the bluer a star, the hotter it is. Measuring the relationship between observed colors and brightnesses of stars helps astronomers figure out the evolutionary sequences of stars.

It turns out that you can do almost the exact same thing for galaxies. Except this time, the color of a galaxy is a measure of how quickly it produces stars, called its star formation rate. This is because hot (and therefore blue) stars are extremely bright, despite being relatively rare, so they can make a whole galaxy appear blue. And such hot stars don’t live for long, so if a galaxy has these stars it must have formed them very recently. On the other hand, the brightness of a galaxy is roughly correlated with its mass, since a more massive galaxy will generally have more stars and therefore be brighter than a less-massive galaxy.

The color-mass diagram

In the same way we use color-magnitude diagrams to determine how stars evolve, we can use a plot of galaxy color (star formation rate) against mass (brightness) to figure out how galaxies evolve.

Figure 1. A cartoon of a low-redshift color-mass diagram. On the y-axis, “number of stars forming” is the star formation rate, which is correlated with color; on the x-axis, “number of existing stars” is the mass, which is correlated with brightness. [CANDELS Collaboration]

Figure 1 (which is a cartoon that I’ve shamelessly borrowed from this previous Astrobite) shows a few basic trends that emerge from this color-mass plot. There’s a “main sequence” of blue star-forming galaxies, where most galaxies lie. It’s thought that galaxies move from this main sequence to the “red and dead cloud,” made up of red galaxies that aren’t actively forming many stars. Not many galaxies are located in the region between these two areas, sometimes called the “green valley,” suggesting that galaxies don’t stay there for a very long time — so the processes that “quench” (turn off) star formation in blue galaxies must be relatively fast.

These basic trends appear on the color-mass diagram in the nearby universe at z~0 (z is what astronomers call “redshift,” which is both a measure of distance and of the universe’s age). Today’s paper aims to understand the trends on the color-mass diagram at higher redshifts (z~1), when the universe was younger — less than half its current age!

Today’s paper

More specifically, the authors want to answer a few questions:

  1. Do overall trends in the color-mass diagram look different at high-redshift (z~1) than at low-redshift (z~0)?
  2. Do elliptical (what the authors call “bulge-shaped”) and spiral (“disk-shaped”) galaxies follow the same trends on the color-mass diagram?

If the answer to question (1) is “yes,” then we know that the processes that shut off star formation have changed from redshift z~1 to now. And because most bulge-shaped galaxies are thought to be the results of galaxy mergers (although this is up for some debate), answering question (2) would help us figure out how mergers affect galaxy evolution.

To address these questions, the authors first used Hubble Space Telescope images (specifically, the GOODS and CANDELS surveys) to measure the colors of about 5,000 galaxies. Next, they matched the galaxy spectral energy distributions to theoretical models in order to infer their masses. Finally, they fit the galaxy images to different profile shapes to distinguish between “bulge” and “disk” galaxies.

Armed with this data, let’s see if the questions above were answered.

screen-shot-2017-01-22-at-11-38-52-pm

Figure 2. The color-mass diagram at z~1. Note that the y-axis is reversed from Figure 1, so that bluer (higher SFR) galaxies are lower and redder galaxies are higher. This means that the “main sequence” is marked by the contours in the bottom left, while the “red and dead cloud” is marked by the contours in the upper right. Purple points are active galactic nuclei (AGNs). [Powell et al. 2017]

  1. Do overall color-mass trends change with redshift?
    Figure 2 shows the color-mass diagram at z~1. Like Figure 1 (which is a cartoon of the z~0 color-mass diagram), it shows that the “main sequence” and “red and dead cloud” are mostly distinct. The main difference is that there are fewer “red and dead” galaxies than at z~0. This is probably because there hasn’t been as much time since the peak of star formation in the universe (which was at redshifts z~1–3) for galaxies to move away from the main sequence.
  2. Do bulges and disks follow the same color-mass trends?
    Disk galaxies follow pretty much the same trend as the gray points in Figure 2, although the “main sequence” and “red and dead cloud” are not as distinct. In contrast, bulge galaxies (see Figure 3) are mostly clustered in the “red and dead cloud,” with very few galaxies between the cloud and the “main sequence.” This means that disks probably evolve gradually, but bulge galaxies (which are the results of mergers) undergo a much more abrupt quenching process. (Note that this agrees with the results reported in this Astrobite for low redshifts!)

Figure 3. Color-mass diagrams for bulge galaxies. The galaxies are colored by specific star formation rate, which is just star formation rate divided by mass. [Powell et al. 2017]

So we’ve answered all our questions! But the authors note that there are still some unclear results. For instance, they also compare the color-mass trends of active galactic nuclei (AGNs; the purple points in Figure 2) with the trends of inactive galaxies. This is an important question in galaxy evolution, since it’s not clear whether feedback from AGNs helps to trigger or quench star formation. The authors find that AGNs might have some effect on quenching, particularly in mergers, but more data is needed to be certain.

The color-mass diagram has been around for a while — but as today’s paper shows, it’s still an incredibly useful diagnostic tool that can teach us a thing or two about galaxy evolution.

About the author, Mia de los Reyes:

I’m a postgrad student at the University of Cambridge, where I work with Rob Kennicutt to study star formation in galaxies (mostly I make lots of graphs). I did my undergrad at NC State University, which is where I started making graphs. Now, whenever I’m not making graphs, I can usually be found rock climbing or eating baked goods.

NGC 2392

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we will be reposting astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Masses of the Planetary Nebula Central Stars in the Galactic Globular Cluster System from HST Imaging and Spectroscopy
Authors: George H. Jacoby, Orsola De Marco, James Davies, I. Lotarevich, Howard E. Bond, J. Patrick Harrington, Thierry Lanz
First Author’s Institution: Lowell Observatory
Status: Accepted to ApJ, open access

Have you ever looked at something and wondered, “How did that get there?!” Has that something ever been a planetary nebula? Astronomers are scratching their heads over four planetary nebulae that have turned up in the unlikeliest of locations: globular clusters.

We know of thousands of planetary nebulae in the Milky Way and can even study planetary nebulae in other galaxies. So the first question is: why shouldn’t we see them in globular clusters? To understand this, we need to know a little bit more about how planetary nebulae are formed.

Planetary nebulae (PNe, singular PN) form during a fleeting phase in the life cycle of low- and intermediate-mass stars (a good range to remember is 0.8 to 8 times the mass of the Sun; our Sun is likely to become a PN in ~5 billion years). PNe consist of a central white dwarf wreathed in shells of hot diffuse gas ejected during the asymptotic giant branch phase late in the star’s evolution. The gas is ionized by ultraviolet photons from the central star, giving rise to some of the most colorful and beautiful objects in the Universe (see Figure 1). The term “planetary nebula” is an unfortunate misnomer; an overzealous William Herschel, fresh from his discovery of Uranus in 1781, thought the fuzzy greenish object he spied through a telescope bore a striking resemblance to his recent planetary find.

m57_nasa_hst

Figure 1. M57, otherwise known as the Ring Nebula, is an elliptical planetary nebula that can be observed even with a small telescope. [NASA/HST]

In order to create a visible PN, the central star must become hot enough to produce ultraviolet photons to ionize the nebula before it drifts away from the central star and dissipates. Stars with masses less than ~0.8 solar masses are thought not to form visible PNe because by the time the central star becomes hot enough to ionize the nebula, the nebular material has already dispersed. Given the age of the globular clusters investigated in the work (11.6 – 13.2 billion years) and stellar evolution models, stars in these clusters with masses of ~0.8 solar masses should be departing the main sequence. In other words, the turn-off mass is approximately equal to the lower limit for PN formation. If the stars departing the main sequence in globular clusters aren’t massive enough to form PNe, how did the PNe we observe come to be?

The leading theories for how we might be able to see PNe in globular clusters involve interactions between two stars. First, the observed PNe might be the descendants of blue stragglers — main sequence stars with masses higher than the cluster turn-off mass which are thought to be the result of two stars merging. The resultant PN would appear to be the evolutionary product of a single massive star, but the PN central star would be more massive than a typical white dwarf in the cluster. Alternatively, the PNe could result from post-common-envelope binaries. (You can learn more about post-common-envelope binaries and how they relate to PNe here.) The common envelope accelerates the star’s transition between asymptotic giant branch star and white dwarf. As a result, the PN central star can have a mass equal to or less than the typical cluster white dwarf mass.

In this paper, the authors analyzed Hubble Space Telescope observations of the four known globular cluster PNe in order to determine the most likely formation scenario for these objects. Optical images of the four target objects can be seen in Figure 2.

pne

Figure 2. The four PNe investigated in this paper, as seen by the Hubble Space Telescope. From left to right: Ps 1, IRAS 18333, JaFu 1, JaFu 2. [Jacoby et al. 2017]

The authors used stellar evolutionary tracks — models of how the temperature and luminosity evolve after a star leaves the main sequence — to determine the masses of the central stars. Combining the derived central star masses with secondary information such as the morphology of the individual nebulae, they conclude:

  1. Two PNe (first and third in Figure 2) most likely resulted from a merger or mass transfer. However, the masses cannot yet be determined precisely enough to distinguish between the formation scenarios described above.
  2. One PN (far right in Figure 2) shows only weak evidence for a binary interaction. If PNe in globular clusters arise from single stars, it would require a re-evaluation of established evolutionary timescales.
  3. The last object (second from the left in Figure 2) is so bizarre that the authors questioned its membership in the PN class altogether!

With only half of the known four globular cluster PNe requiring some form of binary interaction, we can’t yet invoke binaries as the cause of globular cluster PNe. Despite this, it’s still important to understand the role that binary and multiple star systems play in PN formation because so many stars in the Galaxy fall into the PN progenitor mass range. Do binary systems help shape many PN? Or can single stars put on a spectacular end-of-the-line display without assistance from a companion? Earthlings, five billion years from now, will be waiting to find out.

About the author, Kerrin Hensley:

I am a second year graduate student at Boston University, where I study the upper atmospheres and ionospheres of Venus and Mars. I’m especially interested in how the ionospheres of these planets change as the Sun proceeds through its solar activity cycle and what this can tell us about the ionospheres of planets around other stars. Outside of grad school, you can find me rock climbing, drawing, or exploring Boston.

pulsar bow shock

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we will be reposting astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Hubble Space Telescope detection of the millisecond pulsar J2124–3358 and its far-ultraviolet bow shock nebula
Authors: B. Rangelov, G. G. Pavlov, O. Kargaltsev, A. Reisenegger, S. Guillot, M. van Kerkwijk & C. Reyes
First Author’s Institution: George Washington University
Status: Accepted to ApJ, open access

Pulsars — the rapidly rotating, highly magnetized neutron stars that beam radiation from their magnetic axes — are as mysterious as they are exotic. They’re most often observed at radio frequencies using single-dish telescopes, and they’re sometimes glimpsed in X-ray and gamma-ray bands. Far rarer are pulsar observations at “in-between” frequencies, such as ultraviolet (UV), optical, and infrared (IR) (collectively, UVOIR); in fact, only about a dozen pulsars have been detected this way. However, their study in this frequency range has proved enlightening, as we will see in today’s post.

A pulsar too hot to handle

While one would expect a neutron star to cool with age if an internal heating mechanism does not operate throughout its lifetime, observations of the millisecond pulsar J0437–4715 (an interesting object in its own right) yielded surprising results. In a 2016 study, far-UV observations revealed the 7-billion-year-old pulsar to have a surface temperature of about 2 × 105 K — about 35 times the temperature of the Sun’s photosphere. This finding inspired Rangelov et al. to observe another millisecond pulsar, J2124–3358 (a 3.8-billion-year-old pulsar with a spin period of 4.93 ms), in the far-UV and optical bands using the Hubble Space Telescope (HST).

Because so few pulsars have been studied in these frequency ranges, their spectral energy distributions (SEDs) in this regime are poorly understood. Generally speaking, the spectra of normal, rotation-powered pulsars reveal a nonthermal (not dependent on temperature) component in optical and X-rays caused by electrons and positrons in the pulsar magnetosphere. In the far-UV, some pulsars show a thermal (blackbody) component in their spectra, thought to come from the surface of the cooling object. Analysis of the team’s HST images revealed an SED that is best modeled by a combined nonthermal and thermal spectral fit, with nonthermal emission dominating at optical wavelengths and thermal emission appearing in the far-UV (see Figure 1). If their interpretation is correct, this implies a surface temperature for J2124–3358 that is between 0.5 × 105 and 2.1 × 105 K, which is very much in line with the temperature of J0437–4715. If this proves to be the case, these two measurements will strongly suggest the presence of a heating mechanism in millisecond pulsars. However, various fits using only nonthermal components in the far-UV are still valid, so it is impossible to make an absolute determination of the correct fit.

There are quite a few heating mechanisms that could be invoked to explain these objects’ high temperatures, ranging from the release of stored strain energy from the pulsar’s crust to dark matter annihilation in the pulsar’s interior. More spectral coverage of J2124–3358 is necessary to both check the validity of the nonthermal and thermal combined fit and to get closer to determining more specifically the heating mechanism in play.

Figure 1: thermal and nonthermal combined fit to HST far-UV/optical data for J2124

Figure 1: Thermal (red dashed) and nonthermal (blue dashed) combined spectral fit to HST far-UV/optical data for J2124–3358. The black line signifies the sum of both components. Because there is uncertainty about the nature of the nonthermal component, two possible spectral slopes are shown. [Rangelov et al. 2016]

A (bow) shocking find in the far-UV

Images of J2124–3358 also show the presence of a bow shock, which is an arc-shaped shock that occurs when an object is moving faster than the interstellar medium (ISM) sound speed. J2124–3358 was known before this study to be accompanied by such a shock in H-alpha (Hydrogen transition from n=3 to n=2) filters, for which plenty of neutral hydrogen is required. As a result of the HST observations, J2124–3358 was found to have an (albeit fainter) far-UV shock coincident with the H-alpha shock (see Figure 2). This is only the second such object (after J0437–4715) to show a far-UV bow shock. It is absolutely possible that many pulsars cause bow shocks that don’t emit in H-alpha, but do in other wavelength regimes. Studying these more carefully will yield information about the nature of the ISM.

In order to learn more about the heating mechanisms operating in these objects as well as the bow shocks that sometimes accompany them, many more pulsars will need to be studied using various optical, UV, and IR filters. Studies in the far-UV are only possible with Hubble, so it will be a long time before a sufficient number of objects will be studied at these frequencies in order to make solid conclusions about the nature of such interesting phenomena.

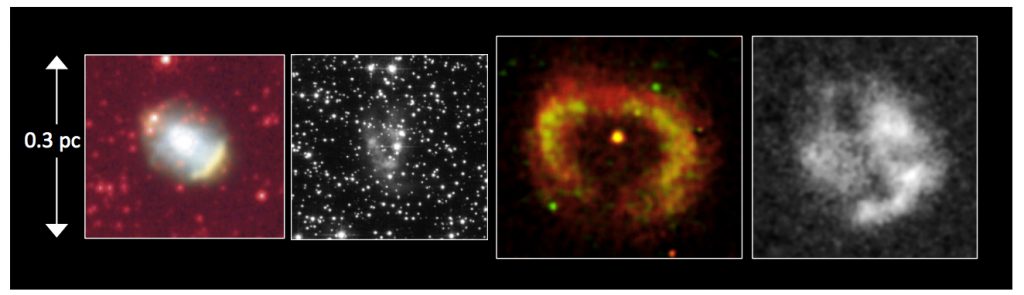
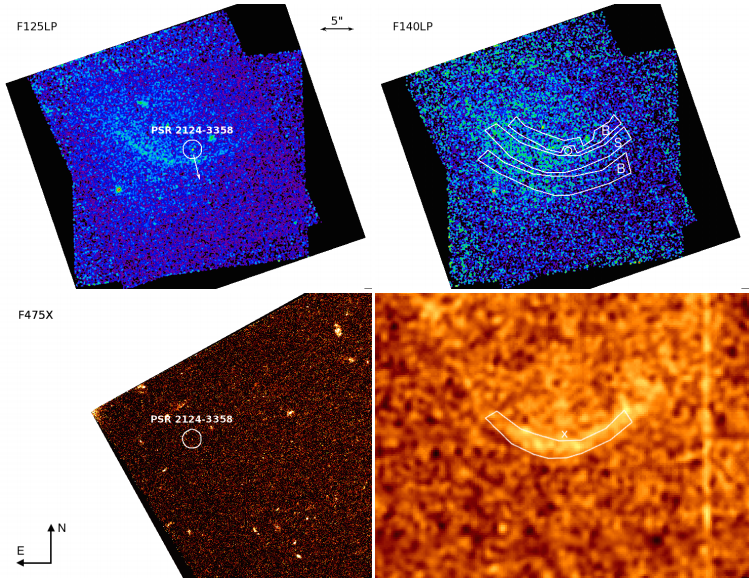Figure 1: New observations from this study using the HST at three different wavelengths are shown in the top (left and right) and bottom left images. The shock is clearly visible in the far-UV using the F125LP filter. The bottom right image shows a previous H-alpha observation of the same pulsar. Figure 1 in the paper.Figure 2: New observations of J2124–3358 from this study using the HST at three different wavelengths are shown in the top (left and right) and bottom left images. The shock is clearly visible in the far-UV using the F125LP filter. The bottom right image shows a previous H-alpha observation of the same pulsar. [Rangelov et al. 2016]

About the author, Thankful Cromartie:

I am a graduate student at the University of Virginia and completed my B.S. in Physics at UNC-Chapel Hill. As a member of the NANOGrav collaboration, my research focuses on millisecond pulsars and how we can use them as precise tools for detecting nanohertz-frequency gravitational waves. Additionally, I use the world’s largest radio telescopes to search for new millisecond pulsars. Outside of research, I enjoy video games, exploring the mountains, traveling to music festivals, and yoga.

Swift

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the partnership between the AAS and astrobites, we will be reposting astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: Discovery of a Transient Gamma-Ray Counterpart to FRB 131104
Authors: J. J. DeLaunay, D. B. Fox, K. Murase, P. Mézáros, A. Keivani, C. Messick, M. A. Mostafa, F. Oikonomou, G. Tešić, and C. F. Turley
First Author’s Institution: Pennsylvania State University
Status: Published in ApJL, open access

The Parkes radio telescope in Australia. [CSIRO]

The Parkes radio telescope in Australia. [CSIRO]

It’s a mysterious case worthy of Sherlock Holmes: seemingly random bursts of radio emission generated from somewhere outside the Milky Way, with no obvious source. These emissions, known as Fast Radio Bursts (FRB), have plagued astronomers over the last several years. They were initially discovered in data archives of the Parkes radio telescope in Australia, popping up as relatively strong radio bursts that lasted only 5 milliseconds. The usual radio bursts we detect are usually repeating, emanating from rapidly spinning neutron stars known as pulsars. This radio signal was all alone.

Even stranger, the signal was dispersed, which means the higher frequency portion of the burst was detected before the lower frequencies. Dispersion is a result of the lower frequency signal being slowed preferentially compared to the high frequencies by the electron clouds between us and the source. Measuring the delay between low and high frequency gives us the distance the signal has traveled. This dispersion indicated that the burst must have originated from very far away — at least 1 Gigaparsec distant. That’s a span of over 3 billion light-years!

Since the archival discovery of FRBs, these sneaky radio bursts have been caught in the act by Parkes telescope, as well as Arecibo in Puerto Rico and the Green Bank Telescope in West Virginia. But until now, there have been no detections in any other wavelengths than radio. In today’s paper, we see for the first time a possible counterpart for an FRB detected in very high energy gamma rays using the Swift telescope. Swift has onboard the Burst Alert Telescope (BAT) that is usually triggered when a gamma-ray burst goes off somewhere in the universe. If typical gamma-ray bursts were responsible for FRBs, then the BAT would have been triggered around the time of an FRB detection, providing almost instantaneous gamma-ray measurements in the same general direction of the the FRB. The authors of today’s paper, however, wondered if perhaps there have been gamma-ray counterparts to the FRBs, but they were not luminous enough to trigger the BAT on Swift. The authors therefore went digging in the archival BAT data, looking for times when Swift just fortuitously happened to be looking in the same direction as an FRB when the radio signal was detected.

FRB

The Swift BAT detection of FRB 131104. The top panel (a) shows the portion of the field of view of the BAT where the gamma-ray counterpart was detected, denoted by the black circle near the top of the image. The x- and y-axes denote the position on the sky in right ascension (RA) and declination (dec), and the color bar shows how well-detected the emission is, in units of signal above the noise. The bottom panel (b) gives the number of photons detected per second as a function of time for gamma rays in the 5–15 keV energy range.

Sure enough, there were four times when an FRB was detected within the same field of view as the BAT on Swift. Of these four possibilities, a gamma-ray counterpart was detected for the FRB 131104 (so named for two digits for the year, month, and date of observation.) This detection is shown in the figure above. This FRB is located 3.2 Gigaparsecs away, which corresponds to a redshift of z ~ 0.55. The reason that the BAT was not triggered for this gamma-ray burst was because it was on the very edge of the detector, illuminating only 2.9% of the telescope’s detector. The authors were very careful to rule out other causes for the gamma rays.

Artist's impression of a magnetar in a young star cluster. [ESO/L. Calçada]

Artist’s impression of a magnetar in a young star cluster. [ESO/L. Calçada]

Of course, there are still unanswered questions about this detection. One mystery is that while the FRB lasted only 5 milliseconds, the emission detected in gamma rays lasted several minutes and released significantly more energy — a billion times more — than was detected in the radio burst. One theory posits that the sources of FRBs are brightly flaring magnetars, which are neutron stars with extremely high magnetic fields. However, if magnetars are the culprit, then the gamma-ray emission should not be nearly that long nor that energetic. On the other hand, FRBs with gamma-ray counterparts could be caused by binary neutron stars spiraling into each other. However, models indicate that we should see only about 25 of these events a year, whereas the inferred rate of FRBs is thought to be on the order of thousands per day.

Suffice it to say, I think this is a mystery that would stump even the great detective Holmes himself (minus the small detail that he wasn’t an astrophysicist.) In some ways, this new gamma-ray counterpart discovery is extremely enlightening, giving clues as to where to look next for the source of FRBs. On the other hand, however, this detection has resulted in even more questions about FRB origins. As is often the case in science, more data are needed! In the future, we should be able to fine tune the threshold for triggering the BAT when the next radio burst goes off, allowing us to catch the FRB and its gamma rays in the act.

About the author, Joanna Bridge:

I am a sixth-year Ph.D. candidate at Pennsylvania State University. I study galaxy evolution via emission lines of samples of galaxies that span the entirety of cosmic history. Outside of science, I love to read fiction and do karaoke, among other things! Follow me on Twitter at @bojibridge.

Hodge 301

Editor’s note: Astrobites is a graduate-student-run organization that digests astrophysical literature for undergraduate students. As part of the new partnership between the AAS and astrobites, we will be reposting astrobites content here at AAS Nova once a week. We hope you enjoy this post from astrobites; the original can be viewed at astrobites.org!

Title: ALMA Reveals Potential Localized Dust Enrichment from Massive Star Clusters in II Zw 40
Authors: S. Michelle Consiglio, Jean L. Turner, Sara Beck, David S. Meier
First Author’s Institution: University of California, Los Angeles
Status: Published in ApJL, open access

Galaxies are recycling centers for gas. Dense gas collapses under gravity to form stars. When massive stars form, they quickly impact their surroundings with intense radiation and mass loss. This enriches the galaxy with heavy elements forged inside the massive stars and becomes the raw material for future star and planet formation. The evolution of the galaxy is determined by this cycle of gas to stars and back.

To measure the lifecycle of gas in galaxies, astronomers use radio telescopes that are sensitive to the three main phases of gas. First, the dense gas where stars form emits light with millimeter-size wavelengths from carbon monoxide (CO). Next, gas ionized by massive stars gives off radio waves when particles collide, in a process called free-free emission. Finally, heavy elements lost by massive stars, which will fuel the next generation of star formation, coalesce to form grains of dust that emit light in the submillimeter regime. With an unmatched angular resolution, the Atacama Large Millimeter/submillimeter Array (ALMA) is the tool of choice for studying the cycle of gas in galaxies.

The authors of today’s paper used ALMA to measure the gas and dust in the nearby galaxy II Zw 40. This small galaxy is forming stars at a prodigious rate. The starlight in the galaxy is dominated by massive stars. The authors investigate the effect of these stars on the gas and dust in the galaxy.

Observations of Gas and Dust

With ALMA, the authors observed three different components of II Zw 40. At a wavelength of 3 mm, the dominant source is free-free emission from ionized gas around the massive star cluster. At 870 µm, after accounting for free-free emission, most of the light is emitted by dust grains. These two components are shown in Figure 1. The peaks of ionized and dust emission are distinct, and the dust emission is localized in several clumps around the cluster.

d65425cd-603b-4d60-82a5-4c330da8319f

Figure 1: The galaxy as seen at 3 mm and 870 µm wavelengths. The 3 mm map (upper right and blue contours) shows the extent of free-free emission from ionized gas around massive stars. Removing the free-free contribution from the total 870 µm emission (upper left) gives a map of dust emission (lower left and red contours). [Consiglio et al. 2016]

Figure 2 shows the dense gas compared to dust emission in II Zw 40. Dense gas is traced by emission from CO molecules. The CO emission is offset from dust emission, with areas of dense gas devoid of dust and vice versa. The authors convert the intensity of CO emission to gas mass using the so-called ‘X-factor’. Dividing the mass in gas and dust, the authors find that the gas-to-dust ratio varies from ~70 to 270 across the galaxy. Not only does the ratio vary, but it’s also low overall — there is more dust per unit gas than the authors expect for a galaxy of this type.
a1b64d1b-ac52-417d-8f3a-42f9841fea33

Figure 2: Dense gas and dust emission in the galaxy. Dense gas is traced by emission from CO molecules (blue map). Dust emission is traced by 870 µm emission (orange contours, same as lower left panel in Figure 1). Note the discrepancy between the peaks of dense gas and dust. [Consiglio et al. 2016]

Clumps of Stardust

To explain the low, variable gas-to-dust ratio and the clumpy structure of the dust emission, the authors propose that massive stars enrich nearby clouds with heavy elements and dust. Because this enrichment is ongoing, more dust is joining the dense gas, lowering the total gas-to-dust ratio. The dust is clumpy because it has not yet mixed with the rest of the galaxy. This enrichment model suggests that dust without associated dense gas came from an older star cluster that is not visible in free-free emission (Figure 1) because it no longer ionizes gas.

Pushing Dust With Light

Take another look at Figure 1, and notice how the peaks of dust emission are all slightly offset from the peak of the ionized gas. In addition to ionizing gas, the intense radiation from massive stars can actually push on dust grains. Acting like a multitude of tiny billiard balls, the photons from bright stars exert radiation pressure on the dust. The dust then drifts relative to the gas, which may explain the offset between gas and dust peaks.

ALMA has revolutionized the study of star formation in galaxies. This paper shows that the cycle from dense gas (traced by CO) to massive stars (3 mm) to dust-rich gas (870 µm) is complex. The massive stars in the galaxy have enriched parts of the galaxy, while other areas remain relatively dust-free. The dust-rich products of stellar evolution are pushed by radiation pressure but have not yet mixed into the galaxy. Future studies of the galactic recycling plant will explain the origin and dispersal of the ingredients needed for planets and (perhaps) life. Cue Carl Sagan.

About the author, Jesse Feddersen:

I am a 4th-year graduate student at Yale, where I work with Héctor Arce on the effect of stellar feedback on nearby molecular clouds. I’m a proud Indiana native (ask me what a Hoosier is), and received my B.S. in astrophysics from Indiana University. If I’m not working, you’ll probably find me on a trail or at a concert somewhere.

1 … 38 39 40 41 42